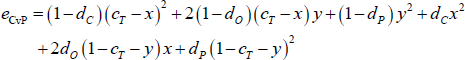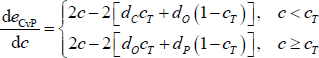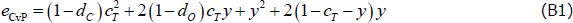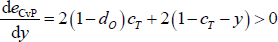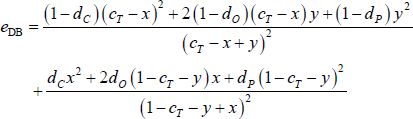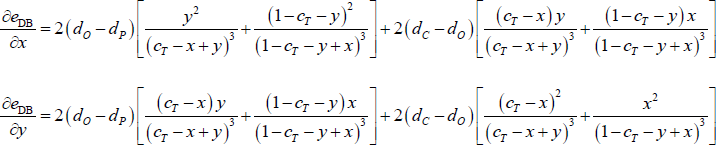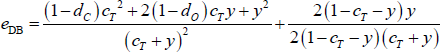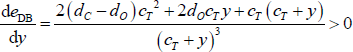RDP 2018-01: A Density-based Estimator of Core/Periphery Network Structures: Analysing the Australian Interbank Market Appendix B: Estimator Accuracy

B.1 Accuracy of the Craig and von Peter (2014) Estimator

Reproducing Equation (4):

If we fix c, and then substitute out y through the identity y ≡ c − c_T + x, then for a given core size the derivative of the error sum with respect to x is:

Since d_C > d_O > d_P (Assumption 3), the above derivative is greater than zero. Therefore, for any given core size, the error-minimising value of x is its smallest possible value. Given the identity y ≡ c − c_T + x, this means x = 0 if c ≥ c_T and x = c_T − c if c < c_T (since y ≥ 0). Using these values for x, the error sum can be written such that the only variable is c. Differentiating e_CvP with respect to c then gives:

With Assumption 3, d_Cc_T + d_O(1 − c_T) > d_Oc_T + d_P(1 − c_T). Therefore, the above derivative at any point in the c < c_T region is lower than at any point in the c ≥ c_T region. Combined with the fact that 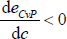when c = 0 and when c = 1, there is a unique global minimum for e_CvP. This unique global minimum depends on the parameter values in the following way:

B.1.1 Error-minimising core size when d_P = 0

When d_P = 0, off-diagonal block errors are possible. However, it requires both true-periphery banks to be incorrectly placed in the core (i.e. y > 0) and all true-core banks to be in the core (i.e. x = 0); if x > 0 then every row/column subset of the off-diagonal blocks will have a non-zero density (see Figure 5). In this boundary scenario, the CvP error function becomes:

Differentiating with respect to y gives:

Therefore, the error function with off-diagonal block errors is bounded below by (1 − d_c). But this is the value of the CvP error function when x = 0 and y = 0. So the CvP estimator will never set x = 0 and y > 0 when d_P = 0, and there will never be any off-diagonal block errors with the CvP estimator.

B.2 Accuracy of the Density-based Estimator

With the simplifying assumptions in Section 4.4, the error function of the DB estimator becomes (i.e. dividing each block in Equation (4) by the number of possible errors within that block):

Differentiating the error function with respect to x and y gives:

Since d_C > d_O > d_P (Assumption 3), the above derivatives are positive for all feasible x and y (i.e. values that ensure c ∈(0,1)). Therefore, the error function is minimised when x = y = 0. This means that c = c_T is the core size that minimises the DB error function.

B.2.1 Error-minimising core size when d_P = 0

Dividing each block in Equation (B1) by the number of possible errors within that block gives:

Differentiating with respect to y gives:

Therefore, the error function with off-diagonal block errors is bounded below by 1 − d_C. But this is the value of the DB error function when x = 0 and y = 0. So the DB estimator will never set x = 0 and y > 0 when d_P = 0, and there will never be any off-diagonal block errors with the DB estimator.