RBA Annual Conference – 2005 Business Cycle Dynamics in OECD Countries: Evidence, Causes and Policy Implications Jean-Philippe Cotis and Jonathan Coppel[1]
Introduction
This paper deals with the interaction between economic policies and the business cycle. It focuses more specifically on the role that improved economic policies may have played in the continuous reduction of price and output fluctuations observed across OECD countries over the past two decades.
As suggested by the recent empirical literature, this issue remains largely unsettled. Some studies document progress achieved over the years in the conduct of monetary policy and conclude, on an ex-ante basis, that it must have contributed to increased price and output stability.[2] Others use econometric analysis to disentangle the respective roles played by improved monetary policies and luck – in the form of smaller and less frequent exogenous shocks – in explaining better outcomes.[3] Overall, they do not support the view that monetary policy had a decisive role to play in reducing price and output instability.
The present work tries to shed some additional and tentative light on these issues by examining recent cross-country stylised facts and adopting a broader policy perspective, extending beyond monetary policy. Focusing on the past five years, and taking a wide cross-country perspective, it suggests that the link between ‘good’ policies and conjunctural stability could be rather strong.
More concretely, a group of countries seems to have nearly ‘extinguished’ the business cycle while enjoying above-average trend growth. This successful group, which includes Australia, Canada, Sweden, the UK and some others, is characterised by monetary policy frameworks of the inflation-targeting type, as well as flexible regulatory frameworks in labour, product and financial markets. These countries are also those that undertook ambitious and comprehensive economic policy reforms over the past couple of decades to break away from a long-standing record of weak growth trends and substantial price and output instability. Therefore, it may not be pure coincidence that indicators for English-speaking and Nordic countries point to a marked reduction in the amplitude of the business cycle.
This spectacular improvement in short- and long-run performance also stands in stark contrast to the more modest changes observed in large continental European countries, both in terms of policy reforms and economic performance. It is indeed striking that, faced with the same sort of negative outside shocks as continental European countries in terms of lost exports and investment, the successful group managed to do a much better job of smoothing consumption and output over the 2001–05 period, while the stance of fiscal and monetary policies was not particularly loose compared to the average in the large euro-area economies. In such a context, the sense of disappointment experienced in continental Europe may stem as much from the persistence of long-standing difficulties as from the realisation that other countries have really succeeded in improving economic performance over the years.
This combination of short-run resilience and, on average, parsimonious use of stabilisation policies strongly suggests that structural flexibility may have been instrumental in offsetting external turbulences. An important source of resilience seems to lie in highly flexible financial markets, in particular in the areas of consumer and mortgage financing that have endowed monetary policy with very strong transmission channels. The flip side of the coin may be, however, that in those resilient countries, neo-Wicksellian monetary policies may be prone to underrating the risks for future price and output stability from asset prices which are out of kilter.
Although this apparently outstanding performance of ‘successful’ countries may be one more streak of luck, it could also be noted that over recent years the international environment has become distinctly less placid. Geopolitical, oil market, as well as trade and exchange rate turbulence may have indeed provided a more stringent and therefore convincing test of the self-stabilising propensities of economies.
Looking at recent comparative evidence there thus seems to be a very strong prima facie case for viewing stabilisation and structural policies as jointly determining long-term growth performance and short-run stability of prices and output. Previous empirical OECD work already provides evidence that good stabilisation policies brought a very significant contribution to long-term growth.[4] And there is an increasing presumption that flexible regulatory frameworks that stimulate potential growth can interact positively with macroeconomic policies to ensure price and output stability.
Moving from descriptive statistics, comparative stylised facts and intuition to harder evidence remains very much of a challenge, however, and motivates work in progress at the OECD. Taking the perspective of ex-ante analysis, it is relatively easy to replicate observed stylised facts and cross-country variations through a calibrated macroeconomic maquette featuring variable degrees of price flexibility in labour, product and financial markets as well as rule-based monetary policies (Section 3.2.1). In terms of ex-post analysis, it has been possible to document, through panel data and comparative analysis, how overly stringent regulatory frameworks are impeding price flexibility in labour, product and financial markets and thus the effectiveness of monetary policy (Section 3.2.2). Finally, ex-post analysis, in the form of SVAR modelling, is currently under way to verify that diverging output trajectories between large continental European countries and members of the ‘successful group’ cannot be explained by differences in shocks or macro-policy stances (Section 3.2.3).
These themes of interaction between economic policy and the business cycle and the role that improved economic policy may have played in the reduction of price and output fluctuations are addressed in the second half of this paper. In the first half, we examine the features of business cycles within countries and the changing degree of business cycle volatility and synchronisation across countries, along with the possible driving forces. The paper is organised as follows. The first section offers a brief overview of the different approaches to the measurement of business cycles. The second section then examines some statistics and stylised facts concerning business cycles in 12 OECD countries over the past 35 years, their degree of volatility and international synchronisation and how it is evolving over time. Such statistics show international convergence towards low output instability, with most spectacular progress achieved by the English-speaking and Nordic countries. A potential trend towards increased synchronisation, stricto sensu, is harder to identify. Section 3 analyses the latest developments in business cycle dynamics, using recent OECD work to examine the interaction with economic policy. Section 4 looks at policy implications.
1. Measuring Business Cycles in OECD Countries
1.1 Defining the business cycle
The business cycle is usually defined as a regular and oscillatory movement in economic output within a specified range of periodicities. The way in which this definition is made operational has evolved over the past half century. In the post-war period, following the seminal work of Burns and Mitchell (1946), cyclical instability was analysed in terms of expansions and contractions in the level of economic activity, typically measured by GDP. These cycles are known as classical business cycles.
This paper focuses only briefly on the classical cycle, because for many OECD economies declines in the level of economic activity are rare events. There are therefore relatively few classical cycles over a 35-year period, making it impossible to make firm inferences regarding the evolution of the size and length of business cycles. An alternative and generally favoured approach to analysing the business cycle is to focus on periods of deviations of output from trend. These episodes, which are more frequent in OECD economies, are known as growth cycles (or deviation cycles). The analysis is concerned with phases of above- and below-trend rates of growth, or movements in the output gap. Even with growth cycles, their frequency is limited over a 35-year period, since each cycle lasts about five years on average.
Moving from classical to growth cycles modifies the meaning of a turning point and phase, both concepts used to describe the morphology of a business cycle. For classical cycles, turning points are reached when output is at a local extremum. Whereas for growth cycles, extrema are defined in terms of output gaps.
Once the turning points are known, the length of each cycle can be identified. In classical cycles, the period between the trough and the peak is the expansion phase and the period between the peak and the trough is the contraction phase. For a growth cycle, the upturn phase is defined as a period when the growth rate is above the long-term trend rate of growth and conversely for the downturn phase. Table 1 provides a taxonomy of the concepts used and their relationship to each other. In practice, applying these definitions literally is difficult, since they imply overly frequent cycles, and thus more sophisticated rules (though still broadly consistent with these stricter definitions) are needed to date the cycle (see Section 2.1).[5]
| Cycle type | Turning points | Phases |
|---|---|---|
| Classical (level of GDP) |
Peaks (P) | P–T contraction |
| Troughs (T) | T–P expansion | |
| Growth (filtered GDP) |
Downturn (D) | D–U low growth rate |
| Upturn (U) | U–D high growth rate |
Before moving to measurement issues, it is important to remember that the classical and growth approaches to the business cycle rely on very different conceptual foundations. While classical cycle analysis is purely descriptive, growth cycle analysis involves a separation between the trend and cyclical components of output that is fraught with statistical and conceptual difficulties (see below).
In this paper, trend output is assumed to be a stochastic unobservable variable, implicitly incorporating technology and other types of supply shocks, while the cyclical component of activity is supposed to be captured by the residual, transitory, component of output. Although this residual component could include, in theory, transitory technology shocks, it is supposed to mainly capture demand-driven fluctuations in output.[6]
A more ‘descriptive’ approach, where trend output is approximated by a set of deterministic trends, may have possibly allowed a more encompassing examination of ‘cyclical’ fluctuations, including those arising from the supply side. However, emphasising the demand side of the business cycle is not without justification, given its centrality in the conduct of stabilisation policies. In short, using ‘real business cycle’ terminology, the paper leaves aside efficient supply-side fluctuations and focuses rather on inefficient demand-driven cyclical fluctuations.
1.2 Measuring the growth cycle in OECD economies
Growth cycles are defined in terms of deviations from trend. The problem, however, is that trend output growth cannot be directly measured. The rate of trend or potential growth is unobservable and has to be inferred from the data. There are many possible approaches to decomposing a series into its trend and cycle components and no single approach can claim to be unequivocally superior (see Box A). Indeed, most of the feasible approaches are ad hoc in the sense that the researcher requires only that the detrending procedure produces a stationary business cycle component, but does not otherwise explicitly specify the statistical characteristics of the business cycle. Hence, the choice of one methodology over another largely hinges on the specific characteristics of the time series and the purpose of the analysis.
In this paper we adopt a band-pass filter to assess the main features of business cycles in OECD countries. This filter is based on the idea that business cycles can be defined as fluctuations of a certain frequency. It eliminates very slow-moving (trend) components and very high-frequency (irregular) components while retaining intermediate (business cycle) components. When applying the filter, the critical frequency band to be allocated to the cycle has to be exogenously determined. Here, we follow Baxter and King (1999) and define the cycle using a uniform low-pass filter to eliminate low-frequency components of more than 32 quarters and a high-pass filter to eliminate high-frequency components of less than 6 quarters. A shortcoming with the filter is that it produces no values for the first and last 12 quarters since it is calculated by a moving average.[7] To compute the output gap for the period 1970 to 2003 we thus first extend our series to 2006 using the OECD's latest short-term economic projections. The data used are quarterly and cover 12 major OECD countries, including Australia.[8]
Box A: Trend-cycle Decomposition Techniques[1]
There is a large literature concerned with the best method of extracting a trend from the data. Within this literature there are three general approaches, based respectively on estimating a structural model of the supply side, using statistical techniques and using survey data.
The first approach derives potential output as the combination of various economic factors. Accordingly, an estimated production function can be used to determine the level of output that would be produced if factor inputs – labour and capital – were fully employed. While widely used by policy-makers for its capacity to reflect the consequences of economic policies on potential output, this approach nonetheless has its limitations: it is not obvious what functional form should be used; taking account of varying qualities of labour and capital may be tricky; and the notion of fully employed labour and capital is not easy to capture, as it depends on the level and intensity of use, which are unobservable and likely to change through time as relative prices evolve. Finally, the production function approach means that technical progress is explicitly modelled, despite not being directly observed, and different estimates of technical progress are likely to lead to somewhat different estimates of the level of potential output.
An alternative, but somewhat related, approach is semi-structural, such as Kalman filters or structural VAR models as developed by Blanchard and Quah (1989). The SVAR approach uses information from the labour market and capacity utilisation to aid in the decomposition of actual output into a permanent trend component (supply) and a temporary cyclical component (demand). The trend is interpreted as a measure of potential output and the cycle as a measure of the output gap. A shortcoming of SVAR techniques is the sensitivity of the results to the identifying assumptions.
The second approach, which does not rely on economic information, is based on statistical or time-series methods. Rather than directly building up an estimate of trend output, they take the data and indirectly identify the trend by decomposing the series into various components. They thus implicitly assume that GDP embodies a long-run equilibrium component and some short-run temporary disturbances along this trend. The problem is that there are a vast number of methods to make this split, each potentially yielding a different dating of the turning points and growth cycle chronologies (Canova 1998 and Quah 1992). The simplest is to fit a linear time trend and assume all deviations from the trend are cyclical. But this is likely to be unreliable during periods of structural change since trend growth itself changes over time.
An equally simple method that avoids a constant trend growth rate is to extrapolate a trend between cyclical peaks, but in practice it can be complicated to implement because it requires a method to identify turning points.
The most frequently used approach is to apply time-series techniques to extract the stochastic trend from GDP data. This allows shocks to aggregate supply to have a permanent effect on output. These techniques use various statistical criteria to identify a trend. Examples include the Hodrick-Prescott filter (HP filter), which is perhaps most common, the band-pass filter (BP filter), or the Beveridge-Nelson decomposition. Each method requires some identifying assumptions, which are often criticised for their arbitrariness and their lack of economic foundations. In practice, the differences across methods are typically small.
The third approach is to construct a measure of capacity utilisation based on business and household survey responses. These responses can then be used to compile a measure of full capacity, with deviations representing cyclical fluctuations. Though this approach is intuitively appealing, experience demonstrates that survey responses do not necessarily reflect aggregate demand pressures, with respondents themselves seemingly finding it hard to disentangle trend from cyclical developments.
All in all, estimating trend output remains an art more than a science and no single approach can be said to be universally superior. The preferred choice remains therefore highly judgemental and dependent on the context and the objective of the work.
2. Features of Business Cycles
2.1 The chronology of classical and growth cycles
Before the characteristics of business cycles can be examined, the first step is to identify the timing of turning points. For that, there is no ideal method, and in practice ad hoc rules of thumb are used, with the results possibly driven by the dating algorithm. This section of the paper follows Harding (2003), by using a more transparent version of the Bry and Boschan (1971) algorithm for dating classical business cycles. The algorithm inevitably involves an element of judgement in terms of the restrictions imposed. These relate to the minimum duration of a cycle to avoid spurious turning points, ensure phases alternate and prevent minor movements in GDP being classified as a cycle.[9] A similar algorithm is also applied to date the upturns and downturns of growth cycles. Appendices A and B show the dates, duration and amplitude of each cycle for the 12 OECD countries included in this study.
2.2 Characteristics of cycles in OECD countries
Based on this method for dating business cycles, Table 2 summarises the main features of classical cycles over the past 34 years. Three points emerge. First, the depth and steepness of contractions appears smaller in the continental European economies than elsewhere, but similarly the vigour of expansion phases is less pronounced. This broadly corresponds to the characterisation of cycles in Europe as ‘U-shaped’ rather than ‘V-shaped’. Second, there is no apparent pattern in the frequency of contraction and expansion phases across countries, although Italy stands out with a higher number of cycles over the period and Canada with relatively few. Third, the average duration of the contraction phase is closely centered on 3½ quarters, while the expansion phase ranges from 12 quarters (Italy) to beyond 30 quarters (France, Japan and the Netherlands).
The ‘U-shaped’ path of European business cycles may reflect the presence of stronger automatic fiscal stabiliser mechanisms linked to generous social expenditure systems which cushion the abruptness of a contraction for a given shock. An explanation for the softer recovery paths in Europe that has sometimes been suggested is that trend growth in continental European economies is slower than in the English-speaking economies, especially over the second half of the sample. This, however, does not appear to be supported by Table 3, which shows the main features of growth cycles, abstracting from trend growth. Indeed, all of the 4 countries where the average amplitude of upturns is below the 12-country average are also euro-area economies.
While the notion of cycles may implicitly convey a sense of regularity and repetition, the features of growth cycles in OECD economies suggest anything but regularity. The length of upturn and downturn phases ranges between 1½ and 3½ years and the steepness, or amplitude, of cycles on average spans a wide range. However, the cumulative movement of cycles, both during upturns and downturns, is relatively similar among countries.
Besides trying to identify country-specific cycles in OECD economies, we examine how the output gap has evolved over time within and across countries. This approach shifts the focus away from narrowly defined cycle analysis and takes a broader view of output fluctuations. On this basis, one feature clearly evident in OECD economies over the past three decades is the drop in the amplitude of output fluctuations, as proxied by the standard deviation of output gaps over approximately nine-year periods since 1970. The fall has been especially marked in Australia, the UK, the US, as well as in Italy, Spain and Sweden and was heavily concentrated over the past decade. Also evident from Table 4 is a tendency for the standard deviation of the output gap among the sampled countries to converge to a lower level. On average over the last nine years, the standard deviation of the output gap is about half what it was during the 1970s.
| Period 1 | Period 2 | Period 3 | Period 4 | |
|---|---|---|---|---|
| Australia | 1.01 | 1.77 | 1.42 | 0.62 |
| Belgium | 1.39 | 1.05 | 1.01 | 0.88 |
| Canada | 1.03 | 1.81 | 1.71 | 0.92 |
| France | 1.01 | 0.75 | 0.91 | 0.89 |
| Germany | 1.41 | 1.17 | 1.26 | 0.71 |
| Italy | 1.92 | 1.14 | 1.00 | 0.62 |
| Japan | 1.97 | 0.95 | 1.19 | 1.13 |
| Netherlands | 0.93 | 1.33 | 0.93 | 0.89 |
| Spain | 1.51 | 0.59 | 1.26 | 0.63 |
| Sweden | 1.61 | 0.98 | 1.79 | 0.88 |
| UK | 1.62 | 1.55 | 1.51 | 0.41 |
| US | 1.91 | 1.89 | 1.02 | 0.96 |
| Euro area | 1.20 | 0.85 | 0.94 | 0.68 |
|
Note: Each period covers 35 quarters – Period 1: 1970:Q1–1978:Q3; Period 2: 1978:Q4–1987:Q2; Period 3: 1987:Q3–1996:Q1; Period 4: 1996:Q2–2004:Q4 Sources: OECD Economic Outlook No 77 database; authors' calculations |
||||
The tendency for the amplitude of the output gap to decline has been associated ex post with reduced volatility of domestic demand as well as a smaller dampening influence from external trade (Table 5). Taken in isolation, the declining contribution of trade to more stable GDP may look paradoxical, in a context where trade openness has continuously increased over the past 35 years. This modest contribution to economic stabilisation may signal, however, that in many countries domestic demand proved less volatile and less likely to trigger equilibrating trade flows. Increased domestic demand stability may partly reflect, in turn, improvements in the conduct of stabilisation policies, with monetary policy in particular putting a stronger emphasis on low and stable inflation. Moreover, as the relative size of the service sector has increased, and with technological innovations improving inventory management, the importance of stock-building to the cycle is less than it used to be.
| Total output gap variance | Contribution from total domestic demand |
Contribution from trade |
Residual | ||
|---|---|---|---|---|---|
| Australia | Period 1 | na | na | na | na |
| Period 2 | 3.14 | 3.70 | −1.52 | 0.96 | |
| Period 3 | 1.82 | 4.39 | −3.40 | 0.83 | |
| Period 4 | 0.38 | 2.64 | −1.96 | −0.31 | |
| Belgium | Period 1 | 1.94 | 3.02 | −0.38 | −0.70 |
| Period 2 | 1.11 | 3.04 | −1.96 | 0.03 | |
| Period 3 | 0.98 | 1.14 | −0.11 | −0.04 | |
| Period 4 | 0.78 | 0.91 | −0.11 | −0.01 | |
| Canada | Period 1 | 1.05 | 2.55 | −1.64 | 0.14 |
| Period 2 | 3.27 | 6.96 | −4.05 | 0.37 | |
| Period 3 | 2.77 | 2.99 | −0.84 | 0.63 | |
| Period 4 | 0.85 | 1.11 | −0.43 | 0.17 | |
| France | Period 1 | 1.03 | 1.90 | −0.90 | 0.03 |
| Period 2 | 0.56 | 0.94 | −0.33 | −0.04 | |
| Period 3 | 0.84 | 1.03 | −0.26 | 0.07 | |
| Period 4 | 0.79 | 1.09 | −0.43 | 0.13 | |
| Germany | Period 1 | 2.09 | 2.91 | −0.62 | −0.20 |
| Period 2 | 1.37 | 3.11 | −1.57 | −0.17 | |
| Period 3 | 1.64 | 1.04 | 0.26 | 0.34 | |
| Period 4 | 0.50 | 1.02 | −0.56 | 0.04 | |
| Italy | Period 1 | 3.70 | 5.86 | −2.66 | 0.51 |
| Period 2 | 1.30 | 2.66 | −1.52 | 0.15 | |
| Period 3 | 0.90 | 2.67 | −2.10 | 0.33 | |
| Period 4 | 0.39 | 0.52 | −0.18 | 0.04 | |
| Japan | Period 1 | 3.88 | 22.41 | −3.09 | −15.44 |
| Period 2 | 0.91 | 4.65 | −0.84 | −2.90 | |
| Period 3 | 1.43 | 4.57 | −0.59 | −2.56 | |
| Period 4 | 1.27 | 1.31 | −0.36 | 0.32 | |
| Netherlands | Period 1 | 0.86 | 2.83 | 4.02 | −6.00 |
| Period 2 | 1.77 | 3.65 | 3.99 | −5.87 | |
| Period 3 | 0.82 | 1.73 | 3.03 | −3.94 | |
| Period 4 | 0.79 | 0.66 | 2.84 | −2.71 | |
| Spain | Period 1 | 2.27 | 3.24 | −1.38 | 0.41 |
| Period 2 | 0.35 | 1.51 | −1.14 | −0.02 | |
| Period 3 | 1.42 | 3.33 | −2.32 | 0.41 | |
| Period 4 | 0.39 | 1.10 | −0.59 | −0.11 | |
| Sweden | Period 1 | 2.58 | 5.27 | −2.01 | −0.67 |
| Period 2 | 0.96 | 1.78 | −1.15 | 0.33 | |
| Period 3 | 2.86 | 3.55 | −1.35 | 0.66 | |
| Period 4 | 0.78 | 1.18 | −0.61 | 0.21 | |
| UK | Period 1 | 2.62 | 2.82 | −1.13 | 0.92 |
| Period 2 | 2.39 | 2.51 | −0.97 | 0.84 | |
| Period 3 | 2.24 | 3.54 | −1.66 | 0.36 | |
| Period 4 | 0.17 | 0.34 | −0.11 | −0.06 | |
| US | Period 1 | 3.66 | 5.28 | −0.94 | −0.68 |
| Period 2 | 3.56 | 5.14 | −0.92 | −0.65 | |
| Period 3 | 0.95 | 1.31 | −0.23 | −0.13 | |
| Period 4 | 0.92 | 1.25 | 0.18 | −0.52 | |
|
Notes: Each period covers 35 quarters – Period 1: 1970:Q1–1978:Q3;
Period 2: 1978:Q4–1987:Q2; Period 3: 1987:Q3–1996:Q1;
Period 4: 1996:Q2–2004:Q4.
For Germany, Period 1 begins with 1971:Q1. Sources: OECD Economic Outlook No 77 database; authors' calculations |
|||||
2.3 Cross-country business cycle relationships in selected OECD countries
OECD economies have become increasingly integrated over the past half century, as trade and investment agreements reduced barriers and improved the climate for cross-border commerce. Today, trade openness in the OECD area is more than double the level in 1960 and foreign direct investment flows have soared. Altogether, this might be expected to result in more similar cycles across countries in terms of their intensity, duration and timing.
However, economic theory is not conclusive about the impact of increased trade on the degree of business cycle synchronisation. Very often, international trade linkages generate both demand- and supply-side spillovers across countries. For example, on the demand side an investment or consumption boom in one country can generate increased demand for imports, boosting other economies. Through these spillovers increased trade linkages result in more highly correlated business cycles. But business cycle co-movement could weaken in cases where increased trade is associated with increased inter-industry specialisation across countries and when industry-specific shocks are important in driving business cycles.[10]
Of course, there are reasons why business cycles do not move in tandem despite increased global integration. Some economies are more susceptible to shocks than others. For instance, economies that are well endowed with commodities, such as Australia, tend to experience greater variation in prices than economies specialised in services, and are therefore more susceptible to wider cyclical movements. Moreover, even if a shock is transmitted internationally, differences in the domestic structure of economies matter. How quickly and at what cost an economy is able to absorb the shock varies, depending on the structure and policy environment (see Section 3). Put succinctly, the degree to which the business cycle has become synchronised across OECD economies is intrinsically an empirical issue.
In this section of the paper, we therefore examine the statistical evidence for growth cycle synchronisation, looking at three aspects: the timing of growth cycle turning points across countries; the length of time cycles are in a similar phase with the cycle in the US; and the similarity of cycles with respect to the intensity of output co-movement across countries.
2.3.1 The timing of the most recent cycle was closely synchronised
The simplest way to approach business cycle synchronisation is to compare turning point dates across OECD countries. This can be achieved, based on the chronology of growth cycles, by examining the density of national turning points at each point in time (Figure 1). A series of closely grouped turning points is indicative of synchronisation. On this basis, there is no clear pattern toward greater or less synchronisation in the timing of turning points. The one possible exception is the most recent downturn in 2001, which was prompted by a global shock. The recovery phase was also tightly grouped, though not all countries in the sample participated in the recovery. Section 3.1 examines the nature of the most recent cycle compared with earlier ones and the differences in the forces driving recoveries across countries.

2.3.2 The duration of phase synchronisation with the US varies widely
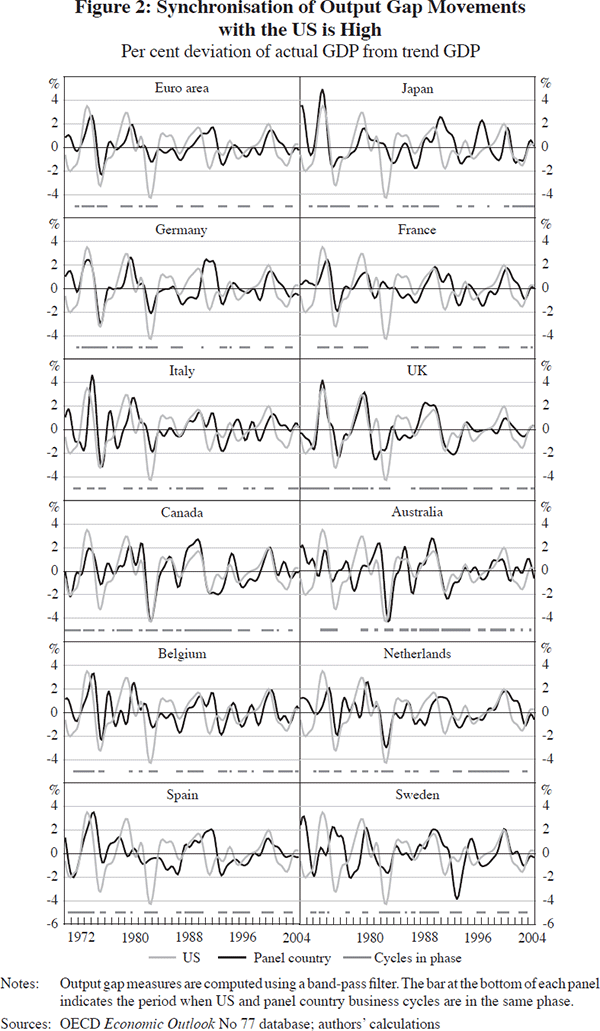
Another aspect of business cycle synchronisation is the proportion of time two cycles are in the same phase. Figure 2 plots the output gap in each country as well as for the US. The bar at the bottom of each panel indicates periods when the two output gaps move in the same direction. What is evident from the graphs is the higher proportion of time that Australia (62 per cent), Canada (75 per cent) and the UK (76 per cent) are in the same phase over the period 1970 to 2004, compared with the euro-area countries (56 per cent). The same calculations show that individual euro-area countries are much more often in phase with the euro area than the US (not shown). In both cases, there is no clear-cut trend towards increased synchronisation of phases over time.
We also examine whether the above stylised facts are corroborated using the statistical framework suggested by Harding and Pagan (2002). They propose examining the degree of concordance between two cycles using the measure:
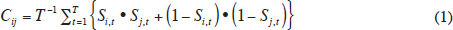
where Si,t represents the business cycle phase of country i at time t (1 represents expansion, 0 contraction), Sj,t is defined similarly for country j and T is the sample size. The measure thus ranges between 0 (business cycles are mirror-images of each other) and 1 (perfect synchronisation). The values in Table 6 in general closely mirror the degree of phase synchronisation shown in Figure 2 and in most cases the concordance statistic is significant.[11] The average degree of concordance suggests the output gaps in these countries move in the same phase 64 per cent of the time, a result which is robust to different sample periods.
2.3.3 The intensity of cycle synchronisation
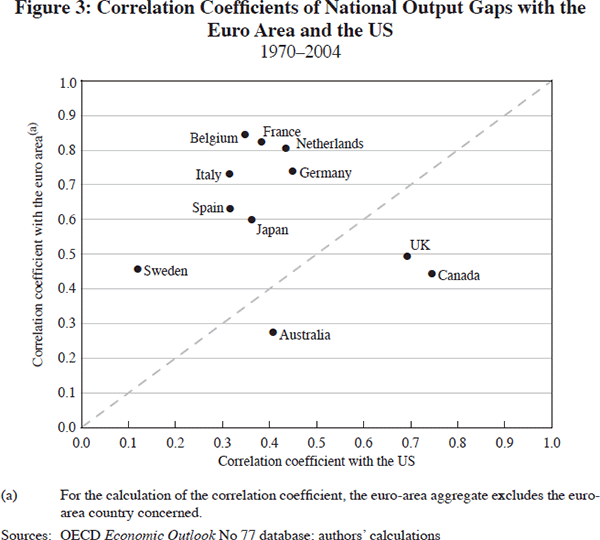
In addition to the timing and direction of bilateral movements in output gaps, the intensity of cycle co-movement matters, especially for policy-makers within a common currency area. In this respect, the size of the bilateral correlation coefficient between the output gap in one country and in another is a crude measure of the intensity of cyclical co-movement across countries. In our calculations, the average bilateral correlation among the countries in this study between 1970 and 2004 is 0.5. This likely understates the extent of cross-border business cycle linkages since the transmission of shocks from one country to another involves lags that are not captured, even for filtered series based on a moving average technique, with contemporaneous bilateral correlations.
Generally, the euro-area countries are more highly correlated with the rest of the euro area than the US. In contrast, Australia and Canada's cycles are relatively more closely synchronised with the US (Figure 3). The UK's greater correlation with the US than the euro area appears puzzling, given the country's close economic ties with the euro area. However, the correlation coefficient mixes characteristics of duration and amplitude into one measure and common shifts in amplitude may be hard to interpret in terms of diffusion and propagation of output fluctuations. An example of such ambiguity may occur when for autonomous reasons – such as universally improved stabilisation policies – countries share a common trend of decreasing output volatility.
The above measures provide a sense of business cycle convergence on average over the period. But they are not well suited to gauge whether synchronisation – in the sense of propagation – has risen over time. Stronger propagation seems likely, however, not least given the increased size of household and corporate balance sheets, with assets whose prices are determined in world markets. One proxy for measuring the changing degree of cycle synchronisation is to examine how the standard deviation of output gaps across countries has evolved. If this measure were consistently zero over time, it would indicate that business cycles in the 12 countries in this study have the same timing and amplitude. On this basis, there is certainly a clear trend towards less divergent cycles over time (Figure 4, top panel).
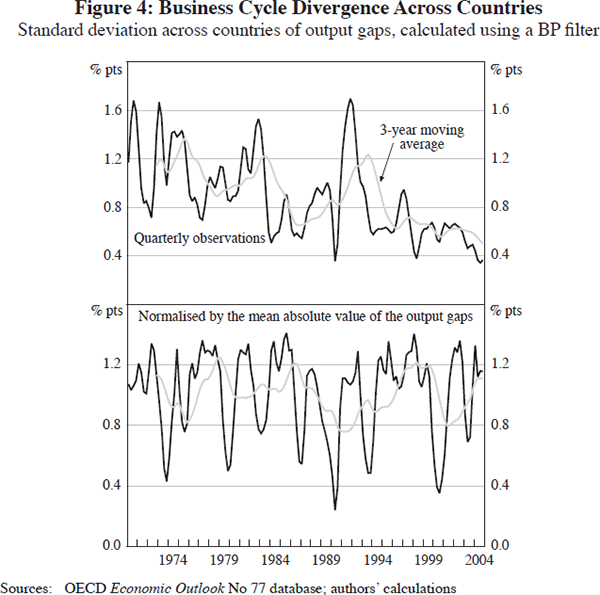
However, since other measures of cyclical convergence (timing of turning points, proportion of time in the same phase of the cycle) do not suggest a clear-cut trend toward increased synchronisation, the reduction in output gap dispersion is also likely to reflect the fact that output gaps on average have become smaller over time. Indeed, when the standard deviation of output gaps across countries is normalised by the average absolute value of the gap to control for the effect of smaller gaps there is no clear downward trend over time (Figure 4, bottom panel).
In summary, these statistics indicate that the amplitude of cycles has diminished over the past 35 years. There has perhaps also been a slight tendency towards fewer and longer growth cycles. Regarding the synchronicity of cycles among the countries examined in this paper, there is a high degree of co-movement in the cycle phase and in the average intensity of co-movement. However, cycle turning points display limited synchronicity and while overall there appears to be a trend towards increased convergence, this seems at least partially linked to the reduced amplitude of cycles in individual countries.
3. Forces Bearing on OECD Business Cycle Dynamics
3.1 Sources of divergence in the current cycle
What is striking with the volatility statistics discussed above is that they do not clearly suggest that the current characteristics of the cycle are notably different across OECD countries. This is despite the now widespread perception that a group of ‘successful’ countries (Australia, Canada, Ireland, New Zealand, the Nordic countries and the UK) did much better than average to weather the 2001 global slowdown, while large continental European countries seem mired in a low activity trap. Such a discrepancy may reflect the difficulty of using statistical filters to distinguish between persistently weak demand and lower trend output, especially at the end of samples. By contrast, volatility statistics computed with OECD traditional production function-based trend output yield a somewhat different picture, and are closer to intuition (Figure 5).
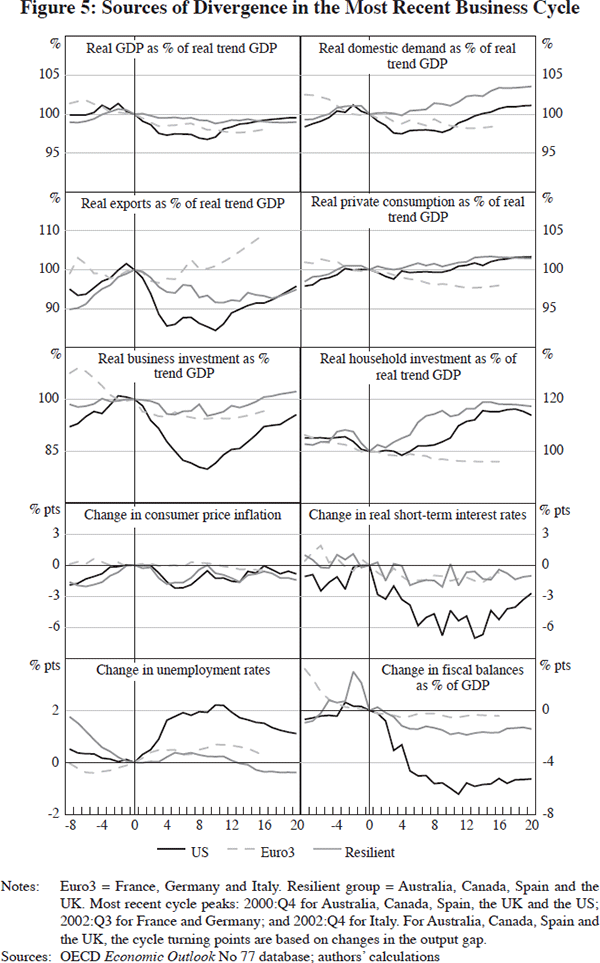
This picture is one of distinct resilience (that is, avoiding long periods away from equilibrium following negative shocks) in the successful group in reaction to the 2001 slowdown. Even though the downturn in all countries was to a large extent prompted by a worldwide demand shock, related to the bursting of bubbles in equity prices and over-investment in ITC equipment, growth relative to trend barely slowed in Australia, Canada, Spain, the UK and some others, whereas the large continental European economies, and hence the euro area as a whole, faced a protracted slowdown. Furthermore, the pace of recovery remains more subdued in the euro area, with the output gap projected to widen further, before starting to close very slowly over the next two years.
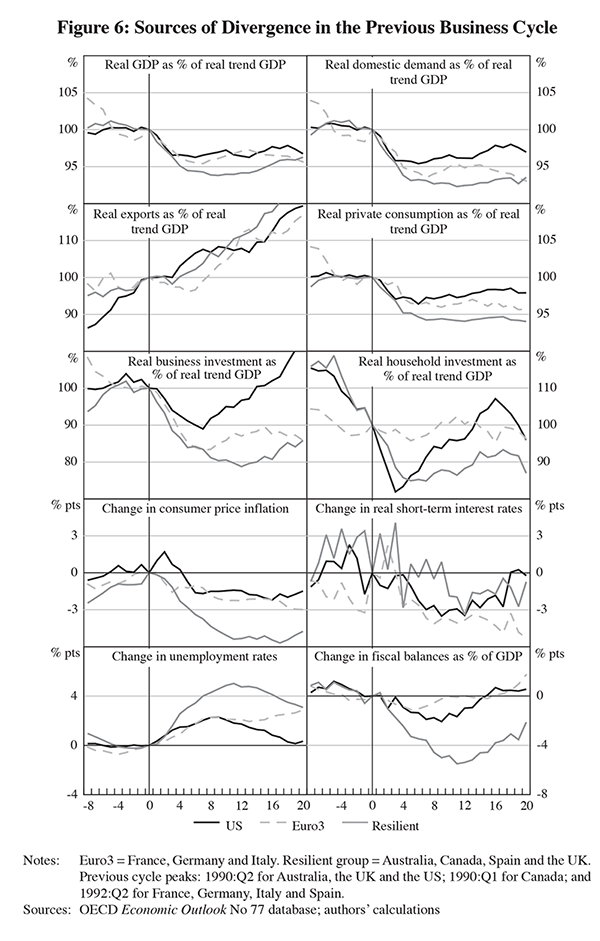
The current situation, with the English-speaking and Nordic countries faring well, stands in stark contrast with the experience of previous slowdowns when these same economies showed fragility during the slowdown and a lack of responsiveness in the upswing (Figure 6). On the contrary, developments in the euro area are similar to previous cycles, suggesting that its relatively lower degree of resilience does not represent an entirely new phenomenon.
A notable difference across country groups in the current cycle has been the behaviour of private consumption and residential investment. In stark contrast with previous episodes, these have shown strength both in the US and in the successful economies, offsetting weakness in the more externally exposed sectors. In contrast, household demand in the euro area failed to buffer the slowdown and support recovery, in line with past experience. Moreover, it appears that these differences can only be partly attributed to disparities in the stance of macroeconomic policy, since a similar aggregate response of monetary and, to a somewhat lesser degree, fiscal policies was observed in both groups of countries (see Figure 5, lower two right-hand panels).[12] This suggests that more fundamental or structural factors are behind divergences in the capacity of the economies to absorb and recover from shocks, including differences in the effectiveness of macroeconomic policies, especially through their influence on domestic demand. The following section examines some of the underlying causes of those differences in the capacity to absorb adverse shocks and speedily recover in their aftermath.
3.2 Why did successful economies become more resilient?
3.2.1 A hypothesis that attributes strong resilience to good structural policies
There are a number of possible linkages between structural policies, growth and resilience that can be invoked to explain how strong long-term growth may also increase short-term adaptability to shocks. These include:
- structural regulatory settings could serve to accelerate the speed of real wage adjustment and to reduce the persistence of unemployment.[13] This will generally lead to shorter deviations of actual output and employment from equilibrium.[14] Also, faster reversals of unemployment to equilibrium reduce the risk that hysteretic effects set in and, therefore, that adverse shocks will permanently lower employment rates;
- regulatory settings, favourable to the development of financial markets, could also contribute to greater consumption smoothing by providing households with better access to credit markets, allowing them to borrow against the least liquid component of their wealth, namely housing.[15] As well, it is likely that flexible and diversified financial markets tend to strengthen the elasticity of domestic and household demand to interest rates (see Section 3.2.2 below);
- flexible product and labour market regulations could speed the recovery process following an adverse shock to the extent that factor reallocation is enhanced. Moreover, by facilitating the process of creative destruction, light regulation may enhance the expansion phase once it takes hold;[16] and
- labour market policies that lead to low structural unemployment and short unemployment duration spells tend to reduce precautionary saving.
To illustrate the effect of flexible labour, product and capital markets and strong monetary policy transmission mechanisms on the degree of resilience of economies, recent OECD work developed a small simulation model with alternative calibrations to replicate economic structures in the US and the euro area.[17] The US model is able to replicate the key properties of the Federal Reserve Board's FRB-US model of the US economy. However, for the euro-area model to display similar properties shown by the European Central Bank's (ECB) Area-Wide Model it was necessary to make adjustments to reflect rigidities in product and labour markets. This was done by lengthening lag structures in price and wage setting and by reducing the impact that any disequilibria have on behaviour. Once calibrated to capture the general workings of the US and euro-area economies, these maquettes can be used to simulate the economic consequences of various shocks. The results broadly suggest that an economy characterised by rigidities tends to be less resilient.
3.2.2 OECD empirical work tentatively supports a relationship between price rigidities and regulatory settings
There is empirical support for the notion that structural policies and institutions in the euro area prolong adjustment and bear adversely on the effectiveness of monetary policy. Concerning, for example, the length of time to adjust to a shock, OECD work has examined why consumer price inflation in the euro area has remained persistently above the ECB's 2 per cent objective even through periods when the output gap was clearly negative.[18] In contrast, prices seem to adjust upwards in a normal manner when capacity constraints are evident.
The responsiveness of prices to output developments was thus explored by estimating an asymmetric Philips curve for a panel of 17 OECD countries, including various non-euro-area economies. Apart from linking inflation to a measure of inflation expectations and the output gap,[19] the model included an interaction term with the output gap to capture the effects of structural rigidities on the cycle. The rigidity indicators used in the regressions were the strength of employment protection legislation and the tightness of product market regulations.[20] The model was estimated with quarterly data over the period 1985 to 2004 using Panel Ordinary Least Squares.
The main result from the analysis is a statistically significant link between more rigid regulatory settings and a weaker response of prices to a negative output gap. Since the euro-area countries score higher on these measures of structural rigidity than the English-speaking countries in the sample, the simulated response of inflation to a widening negative output gap is much weaker in most of the euro area (Table 7).
| Structural indicator used in the regression | ||
|---|---|---|
| Employment protection legislation | Product market regulation | |
| Euro-area countries | ||
| Austria | 0.1 | 0.2 |
| Belgium | 0.4 | 0.2 |
| Finland | 0.2 | 0.3 |
| France | 0.2 | 0.1 |
| Germany | 0.1 | 0.3 |
| Italy | 0.4 | 0.1 |
| Netherlands | 0.0 | 0.2 |
| Spain | 0.0 | 0.2 |
| Other countries | ||
| Australia | 0.5 | 0.3 |
| Canada | 0.5 | 0.4 |
| Denmark | 0.4 | 0.2 |
| Japan | 0.2 | 0.3 |
| New Zealand | 0.5 | 0.3 |
| Norway | 0.0 | 0.2 |
| Sweden | 0.1 | 0.3 |
| UK | 0.6 | 0.4 |
| US | 0.8 | 0.4 |
|
(a) Inflation is measured as the annualised quarterly change in the consumer price index. The results shown here are based on the coefficients drawn from regressing inflation on the previous period output gap, on its interaction with the corresponding rigidity index, on expected inflation and on other variables. Sources: The sources for the data and indicators underlying the calculations are described in Cournède et al (2005). |
||
This result implies that the sacrifice ratio in the ‘rigid’ euro area is larger than in the ‘flexible’ English-speaking countries. Another dimension to resilience, also influencing the size of the sacrifice ratio, is the speed and magnitude with which monetary policy responses to shocks are transmitted through economies. In this regard, other recent OECD work has examined whether the structure of housing and mortgage markets influences the effectiveness of monetary policy.[21] The focus on the housing market is not accidental. It is motivated by the stylised fact, observed above, that a source of divergence across countries in the current cycle relates to the behaviour of residential investment.
The study finds a strong linkage from house prices to activity through wealth channels affecting personal consumption, in line with other research.[22] Housing markets are also important in the transmission of monetary policy. A high interest rate sensitivity is beneficial as it implies that monetary policy is more powerful in boosting or damping cyclical fluctuations. But the effects of monetary policy on activity, as measured by the impact of policy-determined interest rate changes on housing market interest rates and then on house prices and wealth, differ considerably across OECD economies. These differences in the size and speed of interaction between housing and the business cycle can be partly traced back to differences in institutional features of housing and mortgage markets, such as the type of mortgage interest rate regime that predominates (that is, floating or fixed) and the costs of refinancing (Table 8). Those countries where the degree of mortgage market ‘completeness’ is high[23] are associated with a larger estimated long-term marginal propensity to consume out of housing wealth. This suggests that the mortgage market is pivotal in translating house price shocks into spending responses. Indeed, the close relationship of mortgage market ‘completeness’ with real house price–consumption correlations and housing equity withdrawal (HEW) illustrates the crucial role played by the provision of liquidity in connection with housing assets (Figure 7).[24]
| Denmark | France | Germany | Italy | Netherlands | Portugal | Spain | UK | |
|---|---|---|---|---|---|---|---|---|
| LTV ratios | ||||||||
| Typical | 80 | 67 | 67 | 55 | 90 | 83 | 70 | 69 |
| Maximum | 80 | 100 | 80 | 80 | 115 | 90 | 100 | 110 |
| Variety of mortgage products | ||||||||
| Rate structure | ||||||||
| Variable | ** | ** | ** | ** | ** | ** | ** | ** |
| Variable (referenced) | ** | ** | – | ** | ** | ** | ** | ** |
| Discounted | – | ** | – | * | – | – | ** | ** |
| Capped | ** | ** | * | * | ** | – | * | ** |
| Range of fixed terms | ||||||||
| 2–5 | ** | ** | ** | ** | ** | * | * | ** |
| 5–10 | ** | ** | ** | ** | ** | * | * | * |
| 10–20 | ** | ** | ** | * | ** | – | * | * |
| 20+ | ** | * | * | * | * | – | * | – |
| Repayment structures | ||||||||
| Amortising | ** | ** | ** | ** | ** | ** | ** | ** |
| Interest only | * | ** | ** | * | ** | – | – | ** |
| Flexible | * | ** | – | * | ** | – | * | ** |
| Fee-free redemption(a) | ** | – | – | – | – | – | – | * |
| Full-yield maintenance fee | ** | * | ** | * | ** | * | * | * |
| Range of borrower types and mortgage purposes | ||||||||
| Borrower type | ||||||||
| Young household (<30) | ** | * | ** | * | * | ** | ** | ** |
| Older household (>50) | ** | * | * | * | ** | * | * | ** |
| Low equity | – | ** | * | – | * | * | * | ** |
| Self-certify income | – | – | – | – | * | – | * | * |
| Previously bankrupt | * | – | – | – | – | – | – | * |
| Credit impaired | * | * | – | * | * | – | * | ** |
| Self-employed | ** | * | ** | ** | * | ** | ** | ** |
| Government-sponsored | * | ** | * | * | * | ** | * | * |
| Purpose of loan | ||||||||
| Second mortgage | ** | * | ** | ** | ** | ** | ** | ** |
| Overseas holiday homes | ** | ** | * | ** | * | – | – | ** |
| Rental | ** | ** | ** | ** | ** | ** | ** | ** |
| Equity release | ** | – | * | ** | ** | – | * | ** |
| Shared ownership | ** | * | * | * | * | ** | – | ** |
| Mortgage market completeness index(b) | 75 | 72 | 58 | 57 | 79 | 47 | 66 | 86 |
|
Notes: ** readily available; * limited availability; – no availability
‘Readily available’ means that products are actively marketed
with high public awareness. ‘Limited availability’ means that
only a small subset of lenders provide this product, often with additional
conditions. ‘No availability’ means that no lenders surveyed
offered the product. See Mercer Oliver Wyman (2003) for further details
on the sample and criteria of the survey. Source: Mercer Oliver Wyman (2003) |
||||||||
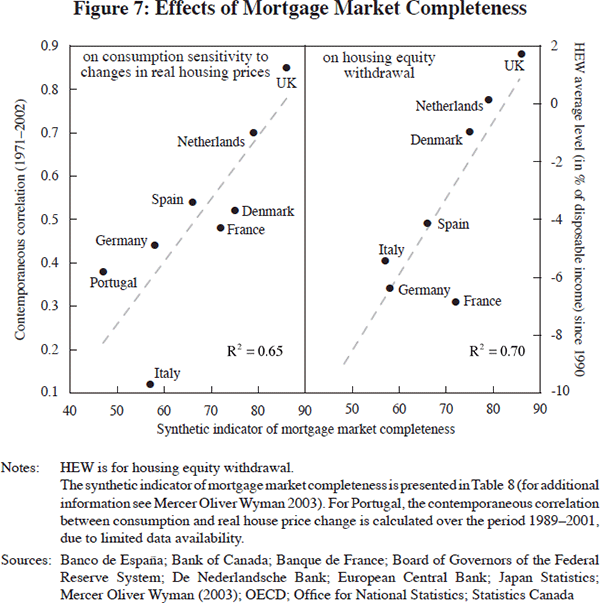
Overall, these studies suggest that structural policies do not only bear on long-term growth, but also on cyclical developments, through two broad channels. The first is by inhibiting or slowing the pace of adjustment to shocks and the second is via weakening the effectiveness of stabilisation policies. However, it could reasonably be argued that the differences across countries in the recent cycle simply reflect more frequent and larger idiosyncratic shocks in the euro area or different policy responses. The next section evaluates this possibility using a methodology that explicitly takes into consideration differences in the source and size of shocks as well as the contribution of macroeconomic policies.
3.2.3 Towards a better understanding of the output costs of differences in resilience
More sophisticated empirical techniques are required to move towards a better understanding of the cost of slow adjustment to shocks, in terms of cumulative output losses, and the role played by structural policies. Initial and ongoing OECD work on this issue is based on the estimation of structural vector-autoregression (SVAR) models for the G7 countries and Spain (because it is a large euro-area country and is also one of the few that appeared resilient to the recent downturn), similar to those recently developed in Buckle et al (2002) and Dungey and Pagan (2000).
The SVAR system allows an analysis of different country responses to standardised shocks. And a cross-country comparison of the responses provides a better indication of the degree of resilience than merely looking at the data since it corrects for the possibility that countries have faced shocks of uneven magnitude and/or different types of shocks. Moreover, the inclusion of equations representing monetary and fiscal policy makes it possible to isolate the impact of automatic and discretionary macroeconomic policy actions on cyclical movements, thereby giving an indication of cross-country differences in resilience that are related to the structural characteristics of countries.
The SVAR approach is equally subject to weakness and limitations. First, the economic interpretation of the estimated shocks is largely dependent on the identifying power of the restrictions imposed on the system; second, the estimation assumes that the underlying structural relationships have been stable over the estimation period; and third, the omission of shocks may affect the results. The specific application of the SVAR models also involves a number of caveats. For instance, it has not been possible to estimate the models taking into consideration possible asymmetric effects between positive and negative gaps in output and other data. Moreover, the gap series have been calculated using a HP filter with a common smoothing parameter across all countries and all series.[25]
Bearing in mind these caveats, the early results from this work tend to confirm the apparent differences in the degree of resilience based on the behaviour of output in the recent cycle. Canada, the UK and the US appear to respond relatively rapidly to all, or most, of the seven shocks analysed, and the cost of shocks in terms of output displacement during the adjustment period is relatively small (Tables 9 and 10).[26] This corroborates prima facie evidence that these economies are more resilient and may in part explain the different pattern of recoveries from the recent cycle.
| Shock to: | World output | Oil prices | Externally focused demand | Domestically focused demand | Inflation | Fiscal policy | Interest rate | Simple average |
|---|---|---|---|---|---|---|---|---|
| Output | ||||||||
| US | 7 | 10 | 16 | 6 | 10 | 4 | 16 | 9.9 |
| Japan | 13 | 20 | 10 | 10 | 20 | 16 | 10 | 14.1 |
| Germany | 13 | 16 | 14 | 10 | 20 | 12 | 16 | 14.4 |
| France | 14 | 18 | 8 | 11 | 11 | 16 | 5 | 11.9 |
| UK | 10 | 15 | 10 | 9 | 13 | 9 | 12 | 11.1 |
| Italy | 13 | 16 | 12 | 7 | 19 | 10 | 10 | 12.4 |
| Canada | 10 | 13 | 10 | 13 | 10 | 5 | 18 | 11.3 |
| Spain | 20 | 13 | 11 | 9 | 9 | 9 | 13 | 12.0 |
| Average adjustment | 13 | 15 | 11 | 9 | 14 | 10 | 13 | 12.1 |
| Domestically focused demand | ||||||||
| US | 12 | 10 | 13 | 6 | 12 | 6 | 10 | 9.9 |
| Japan | 16 | 22 | 13 | 8 | 22 | 12 | 10 | 14.7 |
| Germany | 14 | 14 | 16 | 12 | 22 | 12 | 16 | 15.1 |
| France | 14 | 19 | 10 | 13 | 9 | 16 | 6 | 12.4 |
| UK | 10 | 15 | 10 | 8 | 13 | 9 | 8 | 10.4 |
| Italy | 20 | 12 | 18 | 16 | 16 | 16 | 12 | 15.7 |
| Canada | 8 | 10 | 6 | 14 | 7 | 20 | 20 | 12.1 |
| Spain | 25 | 16 | 12 | 11 | 10 | 10 | 9 | 13.3 |
| Average adjustment | 15 | 15 | 12 | 11 | 14 | 13 | 11 | 13.0 |
| Inflation | ||||||||
| US | 12 | 12 | 14 | 10 | 10 | 12 | 10 | 11.4 |
| Japan | 16 | 20 | 12 | 10 | 22 | 24 | 20 | 17.7 |
| Germany | 19 | 19 | 20 | 14 | 14 | 16 | 16 | 16.9 |
| France | 26 | 16 | 16 | 22 | 22 | 12 | 10 | 17.7 |
| UK | 16 | 10 | 18 | 14 | 13 | 16 | 13 | 14.3 |
| Italy | 22 | 19 | 13 | 19 | 19 | 18 | 18 | 18.3 |
| Canada | 10 | 10 | 16 | 15 | 12 | 12 | 18 | 13.3 |
| Spain | 28 | 18 | 16 | 12 | 11 | 8 | 10 | 14.7 |
| Average adjustment | 19 | 16 | 16 | 15 | 15 | 15 | 14 | 15.5 |
|
Source: OECD |
||||||||
| World output | Oil prices | Externally focused demand | Domestically focused demand | Inflation | Interest rate | Fiscal policy | Simple average absolute value | Average excluding policy shocks | |
|---|---|---|---|---|---|---|---|---|---|
| US | 0.25 | −0.01 | 0.20 | 0.11 | −0.09 | −0.83 | 0.04 | 0.22 | 0.13 |
| Japan | 0.25 | −0.02 | 0.28 | 0.99 | −0.44 | 0.06 | −0.55 | 0.37 | 0.40 |
| Germany | 0.59 | −0.05 | 0.93 | 0.58 | −0.48 | −0.69 | −1.29 | 0.66 | 0.53 |
| France | 0.62 | −0.03 | 0.27 | 0.56 | −0.35 | −0.02 | 0.11 | 0.28 | 0.37 |
| UK | 0.12 | −0.03 | 0.14 | 0.20 | −0.38 | −0.63 | 0.02 | 0.22 | 0.17 |
| Italy | 0.20 | −0.01 | 0.16 | 0.25 | −0.33 | −0.22 | −0.04 | 0.17 | 0.19 |
| Canada | 0.33 | −0.04 | 0.43 | 0.36 | −0.24 | −0.25 | 0.06 | 0.24 | 0.28 |
| Spain | 0.54 | −0.01 | 0.23 | 0.41 | −0.27 | −0.10 | −0.12 | 0.24 | 0.29 |
| Average values | 0.34 | −0.02 | 0.34 | 0.44 | −0.33 | −0.37 | −0.24 | 0.30 | 0.29 |
|
Source: OECD |
|||||||||
In contrast, Germany is found to be a slow adjuster and to incur costly adjustments to most of the shocks, especially in the case of shocks to the globalised parts of the economy. Compared with the US, the adjustment time in Germany is over a year longer on average across shocks. For the other countries included in the study, the speed of output adjustment and the overall cost in terms of cumulative output losses varies significantly across shocks. The shock to inflation (that is, a temporary supply shock) consistently causes the largest negative displacement. The higher relative cumulative output costs seen in the European countries under this shock are consistent with estimates of higher output sacrifice ratios for the euro area, and provide indirect evidence of structural policy settings that hamper adjustment.
The SVAR models were also utilised to decompose past movements in output gaps into their main driving forces.[27] The rationale for doing this is to assess whether relative lack of resilience in the larger euro-area economies, particularly Germany, suggested by the SVAR results is structural in nature (including less favourable macroeconomic policy settings), or whether it can be explained by ‘bad luck’ (larger negative shocks to these economies). The model analysis confirms that the response of monetary and fiscal policies has played an important role in buffering the negative shock in the US in the most recent period. However, even after purging the impact of macroeconomic policy and shocks to the globalised parts of the economy, the growth profile in the big continental European countries over the recent business cycle still seems more sluggish, suggesting intrinsic differences in resilience.
4. Implications for Stabilisation Policies
The evolving nature of the business cycle bears on the capacity of stabilisation policy to smooth prices and output. Specifically, closer international integration limits the effectiveness of active fiscal policy, as a result of leakages through trade and other channels. In the context of a currency union, however, cycle convergence is necessary for effective monetary policy, and indeed is one of the criteria for evaluating the suitability of joining a single-currency zone.
Even though this study did not find strong support for closer synchronisation among the 12 OECD countries considered, it is plausible that ongoing globalisation trends will increase cycle convergence in the future. And it may do so through new channels, such as closer financial market linkages and asset markets whose prices tend to be determined in global, rather than national or local, markets. Accordingly, policy-makers need to remain abreast of new business cycle developments.
Forces acting to change international linkages may also bear on the nature of business cycle dynamics within a country. It is evident, for example, that asset markets now play a bigger role in national economies. This provides both a source of resilience and a risk to stability. A larger value of traditionally less liquid assets in balance sheets (such as housing), together with flexible and innovative financial markets, has arguably made housing markets more responsive to changes in monetary policy through wealth and balance sheet effects. This, of course, means that the magnitude of monetary policy responses needed to modify demand is now smaller than before, and it may also have changed the lags in policy transmission in uncertain ways. These effects need to be better understood.
There is also a danger that the credit and asset price channels of monetary policy transmission have created new potential sources of price and output instability, because asset prices are prone to substantial and prolonged periods of misalignments. Indeed, many of the deepest recessions experienced in OECD countries in the last two decades have been associated with asset price cycles. Obviously, therefore, misalignments in asset prices should be avoided, but it is less obvious how to do so. Here the conventional wisdom that monetary policy responds to the extent that asset price developments bear on demand pressures and thus broader price developments remains, in our view, appropriate. As well, stronger monitoring of systemic risks and flanking policies that bolster prudential regulation and supervision, without harming competition and innovation, have an expanded role to play. This is happening.
More generally, lack of resilience bears on the cost of output deviations from trend and increases the risk of hysteresis and the permanent output losses associated with it. Avoiding sharp and persistent falls in output is therefore of great importance in terms of welfare. Here broader economic policies can help. Removing market rigidities in product and labour markets raises the adaptability of economies to shocks and ultimately economies' potential rates of growth.
5. Conclusions
This paper examines two interrelated aspects of business cycles. The first focuses on the features of business cycles within 12 OECD countries, the degree of international synchronisation and how it is evolving over time. Overall, we find evidence that the severity of cycles has diminished over the past 35 years, reflecting in part improved inventory management techniques, the rising relative importance of services in overall output and more effective monetary policy frameworks for absorbing nominal shocks. Regarding the similarity of cyclical characteristics and the strength of their association across countries, the paper examines a number of aspects, including the timing of growth cycle turning points, the length of time cycles are in a similar phase with the US and the similarity of cycles with respect to the intensity of output co-movement. These measures suggest that business cycle synchronisation is high for the 12 OECD countries included in this study, but the paper is more agnostic concerning the evolution of cycle synchronisation through time. While there appears to be a trend towards increased convergence, this seems at least partially linked to the reduced severity of cycles in individual countries.
These findings are standard and generally supported in the literature. Yet, they do not clearly reveal the recent dichotomy in economic performance between the large euro-area economies, which have experienced a protracted period of excess capacity, and other OECD countries which were better able to absorb the global shock that prompted the slowdown in 2001. The second part of this paper focused more closely on this episode, paying particular attention to the interaction between economic policies and the business cycle. The paper tentatively argues that it is not merely coincidence that the strongly performing OECD economies over the past cycle were also those that undertook ambitious and comprehensive economic policy reforms over the past 20 years. Nor is it sheer ‘good luck’ in the sense of smaller negative shocks to these economies. Rather, recent and ongoing OECD studies based on panel data regressions and SVAR analysis discussed in this paper bolster the presumption that flexible product, labour and financial markets not only stimulate potential growth, but also interact positively with macroeconomic policies to ensure price and output stability. The sense of disappointment experienced in continental Europe may thus stem as much from the persistence of long-standing difficulties as from the realisation that other countries have succeeded in turning their economies around over the years.
Finally, the paper identifies a number of challenges to policy-makers that stem from the evolving features of business cycles within and across countries. Closer international synchronisation will reduce the effectiveness of active fiscal policy, while the expansion of financial markets increases the potency and possibly changes the speed of monetary policy transmission through wealth and balance sheet effects. Policy-makers need to better understand the magnitude of these effects. Through the same channels there is equally a risk of greater price and output instability if asset prices become misaligned. The conventional wisdom of how to respond to asset price misalignments remains in our opinion appropriate, though financial supervision institutions must continue to evolve to take account of financial innovation. Finally, economic policy more generally needs to tackle the lack of resilience to adverse shocks, particularly in the large euro-area countries, by removing micro rigidities in labour and product markets.
Appendix A: Chronology of Classical Cycles
| US | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1973:Q2–1975:Q2 | 8 | −6.78 | −0.85 | 1975:Q2–1979:Q2 | 16 | 6.20 | 0.39 | |
| 1979:Q2–1980:Q3 | 5 | −3.25 | −0.65 | 1980:Q3–1981:Q2 | 3 | 1.28 | 0.43 | |
| 1981:Q2–1982:Q3 | 13 | −5.18 | −0.40 | 1982:Q3–1984:Q3 | 5 | 5.43 | 1.09 | |
| 1984:Q3–1987:Q1 | 9 | −1.74 | −0.19 | 1987:Q1–1989:Q4 | 13 | 2.23 | 0.17 | |
| 1989:Q4–1991:Q3 | 7 | −3.50 | −0.50 | 1991:Q3–1994:Q3 | 12 | 2.36 | 0.20 | |
| 1994:Q3–1995:Q4 | 5 | −1.53 | −0.31 | 1995:Q4–2000:Q2 | 18 | 2.96 | 0.16 | |
| Japan | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q2–1971:Q3 | 3 | −4.18 | −1.39 | 1971:Q3–1973:Q2 | 10 | 5.60 | 0.56 | |
| 1973:Q2–1974:Q4 | 9 | −6.67 | −0.74 | 1974:Q4–1979:Q3 | 16 | 3.34 | 0.21 | |
| 1979:Q3–1983:Q4 | 6 | −2.96 | −0.49 | 1983:Q4–1985:Q3 | 18 | 1.69 | 0.09 | |
| 1985:Q3–1987:Q1 | 4 | −2.12 | −0.53 | 1987:Q1–1990:Q4 | 17 | 4.35 | 0.26 | |
| 1990:Q4–1994:Q1 | 11 | −3.96 | −0.36 | 1994:Q1–1997:Q1 | 14 | 3.67 | 0.26 | |
| 1997:Q1–1999:Q3 | 6 | −3.39 | −0.56 | 1999:Q3–2000:Q4 | 8 | 2.80 | 0.35 | |
| Germany | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q4–1971:Q4 | 4 | −1.77 | −0.44 | 1971:Q4–1973:Q3 | 7 | 2.72 | 0.39 | |
| 1973:Q3–1975:Q2 | 7 | −5.46 | −0.78 | 1975:Q2–1979:Q4 | 18 | 5.70 | 0.32 | |
| 1979:Q4–1982:Q4 | 12 | −4.81 | −0.40 | 1982:Q4–1986:Q1 | 13 | 2.30 | 0.18 | |
| 1986:Q1–1987:Q3 | 6 | −1.53 | −0.26 | 1987:Q3–1991:Q1 | 14 | 3.83 | 0.27 | |
| 1991:Q1–1993:Q3 | 10 | −3.82 | −0.38 | 1993:Q3–1995:Q1 | 6 | 1.82 | 0.30 | |
| 1995:Q1–1999:Q1 | 16 | −1.47 | −0.09 | 1999:Q1–2000:Q3 | 6 | 2.48 | 0.41 | |
| France | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1974:Q1–1975:Q3 | 6 | −4.37 | −0.73 | 1975:Q3–1979:Q4 | 17 | 3.30 | 0.19 | |
| 1979:Q4–1981:Q1 | 5 | −2.17 | −0.43 | 1981:Q1–1982:Q2 | 5 | 1.39 | 0.28 | |
| 1982:Q2–1987:Q2 | 20 | −1.77 | −0.09 | 1987:Q2–1990:Q1 | 11 | 3.04 | 0.28 | |
| 1990:Q1–1991:Q2 | 5 | −1.28 | −0.26 | 1991:Q2–1992:Q1 | 3 | 0.68 | 0.23 | |
| 1992:Q1–1993:Q3 | 6 | −2.67 | −0.44 | 1993:Q3–1995:Q1 | 6 | 1.78 | 0.30 | |
| 1995:Q1–1997:Q1 | 8 | −1.81 | −0.23 | 1997:Q1–2000:Q4 | 15 | 3.20 | 0.21 | |
| Italy | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q3–1972:Q4 | 9 | −3.59 | −0.40 | 1972:Q4–1974:Q1 | 5 | 6.46 | 1.29 | |
| 1974:Q1–1975:Q3 | 6 | −7.83 | −1.31 | 1975:Q3–1976:Q4 | 5 | 4.80 | 0.96 | |
| 1976:Q4–1977:Q4 | 4 | −3.23 | −0.81 | 1977:Q4–1980:Q2 | 10 | 4.32 | 0.43 | |
| 1980:Q2–1983:Q1 | 11 | −4.61 | −0.42 | 1983:Q1–1985:Q3 | 10 | 2.04 | 0.20 | |
| 1985:Q3–1987:Q1 | 6 | −0.72 | −0.12 | 1987:Q1–1990:Q1 | 12 | 2.11 | 0.18 | |
| 1990:Q1–1991:Q1 | 4 | −1.26 | −0.31 | 1991:Q1–1992:Q1 | 4 | 1.13 | 0.28 | |
| 1992:Q1–1993:Q3 | 6 | −3.22 | −0.54 | 1993:Q3–1995:Q4 | 9 | 2.68 | 0.30 | |
| 1995:Q4–1996:Q4 | 4 | −1.75 | −0.44 | 1996:Q4–1997:Q4 | 4 | 1.55 | 0.39 | |
| 1997:Q4–1999:Q1 | 5 | −1.59 | −0.32 | 1999:Q1–2001:Q1 | 8 | 2.26 | 0.28 | |
| UK | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1973:Q2–1975:Q4 | 10 | −6.59 | −0.66 | 1975:Q4–1979:Q3 | 15 | 5.52 | 0.37 | |
| 1979:Q3–1981:Q2 | 7 | −5.70 | −0.81 | 1981:Q2–1983:Q4 | 10 | 2.82 | 0.28 | |
| 1983:Q4–1984:Q4 | 4 | −1.15 | −0.29 | 1984:Q4–1988:Q3 | 15 | 3.16 | 0.21 | |
| 1988:Q3–1992:Q4 | 17 | −4.41 | −0.26 | 1992:Q4–1994:Q4 | 17 | 2.75 | 0.16 | |
| 1994:Q4–1999:Q1 | 17 | −0.95 | −0.06 | 1999:Q1–2000:Q3 | 6 | 1.29 | 0.22 | |
| Canada | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1973:Q3–1975:Q2 | 7 | −3.05 | −0.44 | 1975:Q2–1976:Q2 | 4 | 1.76 | 0.44 | |
| 1976:Q2–1977:Q2 | 4 | −0.93 | −0.23 | 1977:Q2–1979:Q3 | 9 | 2.40 | 0.27 | |
| 1979:Q3–1980:Q3 | 4 | −1.64 | −0.41 | 1980:Q3–1981:Q2 | 3 | 1.88 | 0.63 | |
| 1981:Q2–1982:Q4 | 6 | −6.63 | −1.11 | 1982:Q4–1985:Q3 | 11 | 5.51 | 0.50 | |
| 1985:Q3–1986:Q4 | 5 | −2.58 | −0.52 | 1986:Q4–1989:Q4 | 12 | 4.06 | 0.34 | |
| 1989:Q4–1992:Q4 | 12 | −4.68 | −0.39 | 1992:Q4–1994:Q4 | 8 | 3.47 | 0.43 | |
| 1994:Q4–1996:Q3 | 7 | −2.88 | −0.41 | 1996:Q3–2000:Q3 | 16 | 3.39 | 0.21 | |
| 2000:Q3–2001:Q4 | 5 | −2.35 | −0.47 | 2001:Q4–2002:Q3 | 3 | 1.14 | 0.38 | |
| Australia | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q2–1972:Q4 | 10 | −2.62 | −0.26 | 1972:Q4–1973:Q3 | 3 | 2.15 | 0.72 | |
| 1973:Q3–1974:Q4 | 5 | −2.61 | −0.52 | 1974:Q4–1976:Q4 | 8 | 1.70 | 0.21 | |
| 1976:Q4–1978:Q1 | 5 | −2.54 | −0.51 | 1978:Q1–1981:Q4 | 15 | 4.07 | 0.27 | |
| 1981:Q4–1983:Q1 | 5 | −6.67 | −1.33 | 1983:Q1–1985:Q3 | 10 | 6.41 | 0.64 | |
| 1985:Q3–1986:Q4 | 5 | −3.98 | −0.80 | 1986:Q4–1989:Q3 | 11 | 4.66 | 0.42 | |
| 1989:Q3–1991:Q4 | 9 | −5.12 | −0.57 | 1991:Q4–1996:Q1 | 17 | 2.67 | 0.16 | |
| 1996:Q1–1997:Q2 | 5 | −1.04 | −0.21 | 1997:Q2–2000:Q1 | 11 | 1.73 | 0.16 | |
| Belgium | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q2–1971:Q4 | 6 | −1.95 | −0.33 | 1971:Q4–1974:Q2 | 10 | 4.04 | 0.40 | |
| 1974:Q2–1975:Q3 | 5 | −5.66 | −1.13 | 1975:Q3–1976:Q3 | 4 | 4.19 | 1.05 | |
| 1976:Q3–1977:Q3 | 4 | −3.04 | −0.76 | 1977:Q3–1978:Q2 | 3 | 1.39 | 0.46 | |
| 1978:Q2–1979:Q1 | 3 | −1.21 | −0.40 | 1979:Q1–1980:Q2 | 5 | 3.58 | 0.72 | |
| 1980:Q2–1983:Q1 | 11 | −3.82 | −0.35 | 1983:Q1–1984:Q2 | 5 | 1.19 | 0.24 | |
| 1984:Q2–1987:Q1 | 11 | −1.64 | −0.15 | 1987:Q1–1990:Q1 | 12 | 3.11 | 0.26 | |
| 1990:Q1–1991:Q1 | 5 | −0.86 | −0.17 | 1991:Q1–1991:Q4 | 3 | 1.13 | 0.38 | |
| 1991:Q4–1993:Q2 | 5 | −3.55 | −0.71 | 1993:Q2–1995:Q1 | 7 | 2.70 | 0.39 | |
| 1995:Q1–1996:Q2 | 5 | −2.02 | −0.40 | 1996:Q2–1997:Q4 | 6 | 1.84 | 0.31 | |
| 1997:Q4–1998:Q4 | 4 | −1.40 | −0.35 | 1998:Q4–2000:Q4 | 8 | 2.95 | 0.37 | |
| Netherlands | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q2–1972:Q2 | 8 | −1.41 | −0.18 | 1972:Q2–1974:Q2 | 8 | 2.24 | 0.28 | |
| 1974:Q2–1975:Q3 | 5 | −3.98 | −0.80 | 1975:Q3–1976:Q3 | 4 | 3.20 | 0.80 | |
| 1976:Q3–1977:Q2 | 3 | −1.17 | −0.39 | 1977:Q2–1978:Q1 | 3 | 0.76 | 0.25 | |
| 1978:Q1–1978:Q4 | 3 | −1.34 | −0.45 | 1978:Q4–1980:Q1 | 5 | 3.04 | 0.61 | |
| 1980:Q1–1982:Q4 | 3 | −5.58 | −1.86 | 1982:Q4–1986:Q1 | 21 | 3.90 | 0.19 | |
| 1986:Q1–1988:Q3 | 11 | −1.98 | −0.18 | 1988:Q3–1990:Q4 | 8 | 2.40 | 0.30 | |
| 1990:Q4–1993:Q4 | 12 | −2.60 | −0.22 | 1993:Q4–2000:Q3 | 27 | 3.11 | 0.12 | |
| Spain | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1974:Q2–1975:Q4 | 6 | −4.25 | −0.71 | 1975:Q4–1978:Q1 | 9 | 2.15 | 0.24 | |
| 1978:Q1–1979:Q3 | 6 | −1.58 | −0.26 | 1979:Q3–1980:Q2 | 3 | 0.61 | 0.20 | |
| 1980:Q2–1981:Q4 | 6 | −1.32 | −0.22 | 1981:Q4–1983:Q4 | 8 | 0.52 | 0.06 | |
| 1983:Q4–1986:Q4 | 12 | −1.40 | −0.12 | 1986:Q4–1991:Q4 | 20 | 3.83 | 0.19 | |
| 1991:Q4–1993:Q2 | 6 | −3.92 | −0.65 | 1993:Q2–2000:Q2 | 28 | 3.14 | 0.11 | |
| Sweden | ||||||||
|---|---|---|---|---|---|---|---|---|
| Downturns (upturn to downturn) | Upturns (downturn to upturn) | |||||||
| Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | Period | Duration (quarters) | Amplitude (% points) | Quarterly amplitude (% points) | |
| 1970:Q3–1972:Q1 | 6 | −5.10 | −0.85 | 1972:Q1–1973:Q1 | 4 | 2.44 | 0.61 | |
| 1973:Q1–1973:Q4 | 3 | −0.61 | −0.20 | 1973:Q4–1974:Q4 | 4 | 2.38 | 0.59 | |
| 1974:Q4–1977:Q3 | 11 | −4.32 | −0.39 | 1977:Q3–1979:Q4 | 9 | 4.30 | 0.48 | |
| 1979:Q4–1983:Q1 | 13 | −3.88 | −0.30 | 1983:Q1–1984:Q2 | 5 | 2.31 | 0.46 | |
| 1984:Q2–1986:Q3 | 9 | −1.19 | −0.13 | 1986:Q3–1989:Q4 | 13 | 2.59 | 0.20 | |
| 1989:Q4–1993:Q2 | 14 | −5.95 | −0.42 | 1993:Q2–1995:Q3 | 9 | 5.14 | 0.57 | |
| 1995:Q3–1997:Q2 | 7 | −2.32 | −0.33 | 1997:Q2–2000:Q2 | 12 | 3.14 | 0.26 | |
|
Sources: OECD Economic Outlook No 77; authors' calculations |
||||||||
Footnotes
Jean-Philippe Cotis is the OECD Chief Economist and Jonathan Coppel is a Senior Economist working in Mr Cotis' office. The authors would like to thank conference participants for their very valuable comments and questions. They also greatly benefited from Adrian Pagan's very insightful remarks. The authors would also like to thank Debbie Bloch for her expert statistical assistance, Christophe André for programming the classical cycle dating algorithm and for useful comments and suggestions on an earlier version of this paper together with Jorgen Elmeskov, Vincent Koen and Alain de Serres. The views expressed in this paper are those of the authors and do not necessarily reflect those of the OECD Secretariat or the Organisation's member countries. [1]
See Romer and Romer (2003). [2]
See Stock and Watson (2004). [3]
See OECD (2003b). [4]
From these definitions of classical and growth cycles it follows that classical recessions are always a subset of growth cycle recessions, and there may be multiple classical contraction episodes within a growth cycle recession. While growth cycle downturns tend to lead classical cycle peaks, growth cycle upturns tend to coincide or lag classical cycle troughs. Accordingly, we should expect that high growth rate phases will tend to be shorter-lived than expansion phases and that low growth rate phases will tend to be longer-lived than contraction phases. For more details on the relationship between classical and growth cycles, see Boehm and Liew (1994). [5]
This assumption would not be accepted by proponents of the ‘real business cycle’ school, who tend to interpret the residual component as reflecting short-run supply fluctuations rather than demand-driven ones. [6]
There are critics of the band-pass filter. Harvey and Trimbur (2003) argue that the filter may be inconsistent with some models of trends and cycles. [7]
The resulting output gaps display a broadly similar profile to the OECD output gaps derived from a production function approach and published in the OECD's Analytical Database. Indeed, the impact of differences in detrending methods seems to be felt more strongly on the average level of trend output than on the slope of the trend output series (Claus, Conway and Scott 2000). Since this paper is more concerned with business cycle behaviour over time and across countries, that is, with changes in output gaps rather than output gap levels, the bias implied by a certain filter may not be a significant issue, provided the bias does not vary too much over time or across countries. [8]
When output growth for a single quarter in isolation is negative and large (that is, more than 3 per cent) it is classified as a turning point. [9]
See Kose and Yi (2005) for a discussion on trade linkages and co-movement. [10]
The critical values for the concordance statistic are computed from a formula based on Monte Carlo simulations and reported in McDermott and Scott (2000). To compute the significance levels requires the assumption that the change in the output gap series is normally distributed and the underlying process is a pure random walk, which is not always the case. [11]
If exchange rate developments are taken into consideration, then euro-area monetary conditions hardly changed. [12]
Differences in structural policy and institutions, to the extent that they imply differences in the speed of real wage adjustment across countries, have been identified as one of the reasons why a number of large common shocks in the 1970s and 1980s led to diverse unemployment experiences across countries. See, for example, Bertola, Blau and Kahn (2002), Blanchard and Wolfers (2000), Fitoussi et al (2000) and OECD (1994). [13]
It is equally possible that the deviations are shallower, depending on the source of the shocks. [14]
See, for example, Catte et al (2004). [15]
See, for instance, Bergoeing et al (2002), Caballero and Hammour (2001) and Davis, Haltiwanger and Schuh (1996). [16]
See Drew, Kennedy and Sløk (2004). [17]
See Cournède, Janovskaia and Van den Noord (2005). [18]
The output gap series is from the OECD's Analytical Database. Robustness of the regression results was examined, inter alia, using a univariate estimate of the output gap. [19]
The structural policy variables are defined on a 0–5 scale, with higher values corresponding to more centralised wage coordination or stricter regulation. The degree of concentration in wage bargaining was also examined, but the estimation results are less convincing. [20]
See Catte et al (2004). [21]
See, for example, Pichette and Tremblay (2003) for Canada; Case, Quigley and Shiller (2001) and Benjamin, Chinloy and Jud (2004) for the US; Deutsche Bundesbank (2003) for Germany; OECD (2003a) for the UK; Dvornak and Kohler (2003) for Australia; and Ludwig and Sløk (2004) for a panel of seven countries. [22]
See Mercer Oliver Wyman (2003) for details on the compilation of the index. The index is calculated for the eight countries shown in Table 8. [23]
More generally, ongoing work at the OECD is examining the linkages between financial market development and output growth. [24]
Ongoing work is examining the sensitivity of the results to different detrending approaches for output and other economic series. The preliminary findings presented in this paper, although interesting, should nonetheless be treated with some caution. [25]
The shocks applied are one standard deviation of the estimated structural errors and the reported adjustment times in Table 9 are taken directly from the impulse response profiles. However, because volatility is often exhibited in these profiles during the first year, calculating the initial cycle response times ignores the response profile in the first year. [26]
See OECD (2004) for details on the methodology. While such decompositions can provide valuable information about business cycle dynamics, they are dependent on the underlying, highly simplified model. In practice, economies are subject to a myriad of economic forces, some of which may be idiosyncratic to a country or a specific time period. [27]
Footnotes Box A
For a more detailed discussion, see the Appendix in Cotis, Elmeskov and Mourougane (2005), on which this box is largely based. [1]
References
Baxter M and RG King (1999), ‘Measuring business cycles: approximate band-pass filters for economic time series’, Review of Economics and Statistics, 81(4), pp 575–593.
Benjamin JD, P Chinloy and GD Jud (2004), ‘Real estate versus financial wealth in consumption’, Journal of Real Estate Finance and Economics, 29(3), pp 341–354.
Bergoeing R, PJ Kehoe, TJ Kehoe and R Soto (2002), ‘A decade lost and found: Mexico and Chile in the 1980s’, Review of Economic Dynamics, 5(1), pp 166–205.
Bertola G, FD Blau and LM Kahn (2002), ‘Comparative analysis of labor-market outcomes: lessons for the United States from international long-run evidence’, in AB Krueger and RM Solow (eds), The roaring nineties: can full employment be sustained?, The Russell Sage Foundation and The Century Foundation Press, New York, pp 159–218.
Blanchard OJ and D Quah (1989), ‘The dynamic effects of aggregate demand and supply disturbances’, American Economic Review, 79(4), pp 655–673.
Blanchard OJ and J Wolfers (2000), ‘The role of shocks and institutions in the rise of European unemployment: the aggregate evidence’, Economic Journal, 110(462), pp C1–C33.
Boehm EA and WT Liew (1994), ‘A review of Australia's recent business cycle experiences and a forecast length of the current expansion’, Australian Economic Review, 107, pp 4–19.
Bry G and C Boschan (1971), ‘Cyclical analysis of time series: selected procedures and computer programs’, NBER Technical Working Paper No 20.
Buckle RA, K Kunhong, H Kirkham, N McLellan and J Sharma (2002), ‘A structural VAR model of the New Zealand business cycle’, New Zealand Treasury Working Paper No 02/26.
Burns AF and WC Mitchell (1946), Measuring business cycles, NBER, New York.
Caballero RJ and ML Hammour (2001), ‘Creative destruction and development: institutions, crises and restructuring’, in B Pleskovic and N Stern (eds), Annual World Bank conference on development economics 2000, World Bank (IRBD), Washington DC, pp 213–236.
Canova F (1998), ‘Detrending and business cycle facts’, Journal of Monetary Economics, 41(3), pp 475–512.
Case KE, JM Quigley and RJ Shiller (2001), ‘Comparing wealth effects: the stock market versus the housing market’, NBER Working Paper No 8606.
Catte P, N Girouard, R Price and C André (2004), ‘Housing markets, wealth and the business cycle’, OECD Economics Department Working Paper No 394.
Claus I, P Conway and A Scott (2000), ‘The output gap: measurement, comparisons and assessment’, Reserve Bank of New Zealand Research Paper No 44.
Cotis JP, J Elmeskov and A Mourougane (2005), ‘Estimates of potential output: benefits and pitfalls from a policy perspective’, in L Reichlin (ed), Euro area business cycle: stylized facts and measurement issues, CEPR, London.
Cournède B, A Janovskaia and P van den Noord (2005), ‘Sources of inflation persistence in the euro area’, paper presented at the XVII Villa Mondragone International Economic Seminar on ‘Capitalism and Entrepreneurship Dynamics: Benchmarking Europe's Growth’, CEIS – University of Rome ‘Tor Vergata’, Rome, 6–7 July.
Dalsgaard T, J Elmeskov and CY Park (2002), ‘Ongoing changes in the business cycle: evidence and causes’, OECD Economics Department Working Paper No 315.
Davis SJ, JC Haltiwanger and S Schuh (1996), Job creation and destruction, MIT Press, Cambridge.
Deutsche Bundesbank (2003), ‘Macroeconomic aspects of share price developments’, Monthly Report, 55(3), pp 27–39.
Drew A, M Kennedy and T Sløk (2004), ‘Differences in resilience between the euro-area and US economies’, OECD Economics Department Working Paper No 382.
Dungey M and A Pagan (2000), ‘A structural VAR model of the Australian economy’, Economic Record, 76(235), pp 321–342.
Dvornak N and M Kohler (2003), ‘Housing wealth, stock market wealth and consumption: a panel analysis for Australia’, Reserve Bank of Australia Research Discussion Paper No 2003-07.
Fitoussi JP, D Jestaz, ES Phelps and G Zoega (2000), ‘Roots of the recent recoveries: labor reforms or private sector forces?’, Brookings Papers on Economic Activity, 1, pp 237–311.
Harding D (2003), ‘Towards an econometric foundation for turning-point based analysis of dynamic processes’, paper presented at the 2003 Australasian Meeting of the Econometric Society, Sydney, 9–11 July.
Harding D and A Pagan (2002), ‘Dissecting the cycle: a methodological investigation’, Journal of Monetary Economics, 49(2), pp 365–381.
Harvey AC and TM Trimbur (2003), ‘General model-based filters for extracting cycles and trends in economic time series’, Review of Economics and Statistics, 85(2), pp 244–255.
Kose AM and K-M Yi (2005), ‘Can the standard international business cycle model explain the relation between trade and comovement?’, Federal Reserve Bank of Philadelphia Working Paper No 05-3.
Ludwig A and T Sløk (2004), ‘The relationship between stock prices, house prices and consumption in OECD countries’, Topics in Macroeconomics, 4(1), pp 1114–1140.
McDermott CJ and A Scott (2000), ‘Concordance in business cycles’, IMF Working Paper WP/00/37.
Mercer Oliver Wyman (2003), Study on the financial integration of European mortgage markets, European Mortgage Federation, Brussels.
OECD (1994), The OECD jobs study, evidence and explanations – Part I: Labour market trends and underlying forces of change, OECD, Paris.
OECD (2003a), Economic survey of the United Kingdom, OECD, Paris.
OECD (2003b), The sources of growth in OECD countries, OECD, Paris.
OECD (2004), ‘Differences in economic resilience between G7 countries: evidence from SVAR estimates’, work presented at OECD Working Party No 1.
Pichette L and D Tremblay (2003), ‘Are wealth effects important for Canada?’, Bank of Canada Working Paper No 2003/30.
Qhah D (1992), ‘The relative importance of permanent and transitory components: identification and some theoretical bounds’, Econometrica, 60(1), pp 107–118.
Romer CD and DH Romer (2003), ‘The evolution of economic understanding and postwar stabilization policy’, in Rethinking stabilization policy, A Symposium Sponsored by the Federal Reserve Bank of Kansas City, Federal Reserve Bank of Kansas City, Kansas City, pp 11–78.
Stock JH and MW Watson (2004), ‘Has the business cycle changed? Evidence and explanations’, in Monetary policy and uncertainty: adapting to a changing economy, A Symposium Sponsored by the Federal Reserve Bank of Kansas City, Federal Reserve Bank of Kansas City, Kansas City, pp 9–56.