RBA Annual Conference – 1998 The Macroeconomics of Australian Unemployment Guy Debelle and James Vickery[*]
1. Introduction
The unemployment rate in Australia has risen from less than 2 per cent in the late 1960s to an average of over 8 per cent over the past fifteen years.
In this paper, we examine unemployment from an aggregate perspective and present a framework with which to analyse how the unemployment rate could be permanently lowered from its current level. In doing so, we also discuss the role that monetary policy can play in assisting the adjustment to a lower unemployment rate.
A macroeconomic examination of unemployment obscures much of the rich detail that underpins the labour market. Nevertheless, it enables us to analyse the broad trends that have occurred over the past twenty years on both the demand and supply sides of the labour market. In the next section we examine these trends at an aggregate level. The aggregate unemployment rate has risen as a result of a large increase in labour supply which has not been matched by an equivalent increase in labour demand (employment). Most of the rise in the aggregate unemployment rate occurred in the 1970s, associated with the increase in labour costs at that time. Since the early 1980s, the aggregate unemployment rate has fluctuated with the economic cycle around a relatively constant mean.
In Section 2, we also discuss estimates of the natural rate of unemployment. Similar to the trend in the aggregate unemployment rate, the natural rate rose sharply in the mid 1970s, but has been relatively steady over the past fifteen years fluctuating between 6 and 8 per cent. The adjustment of inflation expectations has played an important role in the movement of the aggregate unemployment rate relative to the natural rate in the 1990s.
An aggregate labour demand and supply curve are at the core of the model described in Section 3 that provides the foundation for the analysis in the paper. These two equations are estimated in Section 4 of the paper. The empirical analysis identifies the effect of real wages and output on labour demand, and the impact of the business cycle on labour supply.
The model presented in Section 3 highlights the importance of the linkage between the level of the aggregate real wage and the unemployment rate. If the aggregate real wage is too high, the unemployment rate will be permanently above its desired level. Consequently, the analysis in Section 5 focuses on the size of the reduction in the unemployment rate that can be achieved by a given reduction in the level of the real wage. It shows that slower real wage growth of 2 per cent below trend for one year could result in a permanent reduction in the unemployment rate of about one percentage point.
The analysis in Section 5 also suggests that monetary policy can play an important role in the transition to a lower unemployment rate. While monetary policy does not affect the natural rate of unemployment or potential output, it can help to reduce the transition time to the lower unemployment rate, thereby reducing the gap between the actual unemployment rate and the natural rate. It can do so by recognising and correctly interpreting the signs of labour market adjustment. The inflation-targeting framework is well suited to this purpose.
2. A Macroeconomic Overview of the Labour Market
Before presenting the theoretical framework and the empirical analysis, we first summarise the trends in the key aggregate labour market variables over the past forty years. Figure 1 suggests that in general, movements in the real cost of employing labour and the cycles in aggregate demand have been associated with fluctuations in employment and unemployment. We investigate these relationships more explicitly in Section 4.

Since 1960, the employment to population ratio has fluctuated around a reasonably constant mean of around 58 per cent, while the unemployment rate has risen by around 6 percentage points. This definitionally implies that the growth in labour demand has not kept pace with the growth in labour supply, particularly over the past 25 years. The driving force behind the increase in labour supply has been the increased participation of females which has more than offset a slow decline in the male participation rate (Borland and Kennedy 1998).
The rise in the participation rate need not have led to a rise in the aggregate unemployment rate, provided there was a decline in the real wage to enable the labour market to absorb the increased supply. This decline would not necessarily be permanent – in a standard trade model, in the long run, the returns paid to factors of production are invariant to the supply of those factors. However, in actuality, as is discussed in the rest of this section, the reverse occurred, namely the real wage increased at the same time as the increase in supply.
Increases in the unemployment rate occurred primarily in three relatively short periods. The first of these was in the mid 1970s and was associated with a sharp rise in labour costs. After the rapid rise in average weekly earnings in 1974 of 28 per cent, real unit labour costs remained at historically high levels until the early 1980s. This rise in labour costs was associated with a five percentage point fall in the employment to population ratio and an increase in the unemployment rate of nearly 4 percentage points.[1]
The unemployment rate increased further around 1982 following the wage push associated with the resources boom of the early 1980s (when average weekly earnings rose at an annual rate of 14 per cent in the two years to June 1982) and as a result of the 1982–83 recession. The wage rise in this case was relatively shortlived, but the unemployment rate rose by 4 percentage points and the employment to population ratio fell by 2 percentage points. In the second half of the 1980s, during the Price and Incomes Accords, real unit labour costs fell below their levels of the early 1970s and employment rose steadily.[2] However, despite the recovery of the employment to population ratio to its level of the early 1970s, the unemployment rate did not fall much below 6 per cent. This was a result of the increase in the participation rate of over 3 percentage points between 1984 and 1990.
Finally, the unemployment rate rose again in the early 1990s following the recession at that time. Subsequently, the employment to population ratio has recovered to around its average level, and the unemployment rate has fallen back to its current level of around 8 per cent.
The sharp rises and the subsequent slow declines in the unemployment rate highlight the costs of large cycles in output and the benefits of maintaining steady growth (Macfarlane 1997). In previous work, we have shown that if the Phillips curve is nonlinear, the more stable the path of economic growth, the lower the average rate of unemployment (Debelle and Vickery 1997). A consistent rate of economic growth close to the trend growth rate of the economy will ensure that the unemployment rate remains close to the natural rate of unemployment through time, thereby lowering the average unemployment rate. On the other hand, a Schumpeterian view of the world would suggest that there are long-run benefits to economic growth from economic cycles. Caballero and Hammour (1996), however, show that even in this case, large volatility in economic growth is likely to be detrimental.
A standard decomposition of the aggregate unemployment rate is into its cyclical and structural components. The structural or natural rate of unemployment is that level associated with a constant and expected inflation rate, given the institutional structure of the economy. Figure 2 shows some estimates of the natural rate in Australia. These estimates are clearly quite divergent, reflecting, inter alia, the different techniques the authors have employed and the different sample periods used in the estimation.
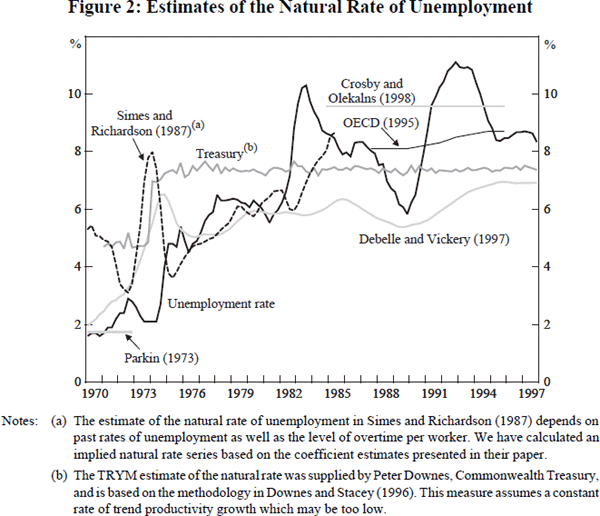
The time series of aggregate unemployment shown in Figure 2 suggests that the natural rate has risen since the early 1970s, but has been relatively constant since the early 1980s. This is supported by Debelle and Vickery's (1997) estimate of the natural rate, which rises sharply in the mid 1970s, and the natural rate series in the TRYM model which has a once-off level shift in 1974 of around 2½ percentage points (Commonwealth Treasury 1996).
The large movements in the natural rate are generally associated with large movements in real wages: the rise in the natural rate in the mid 1970s occurred at the same time as the large shock to the real wage. Similarly, the decline in Debelle and Vickery's estimate of the natural rate in the late 1980s was associated with the moderation in real wage growth.
A number of microeconomic factors are also likely to have influenced the path of the natural rate, including factors that affect a person's willingness to search for a job such as the level of unemployment benefits and the location of job opportunities. However, it has generally proven difficult to directly relate the rise in the natural rate to specific causes, in part because of the difficulty in estimating the natural rate itself. An indirect guide to movements in the natural rate can be obtained by examining factors that have caused shifts in the Beveridge curve which plots the rate of unemployment against the level of job vacancies (Figure 3).[3] The negative slope of the curve is evident, reflecting the fact that in booms, new jobs are being created at a faster pace and there are less unemployed people looking for jobs, while in recessions, the converse is true.
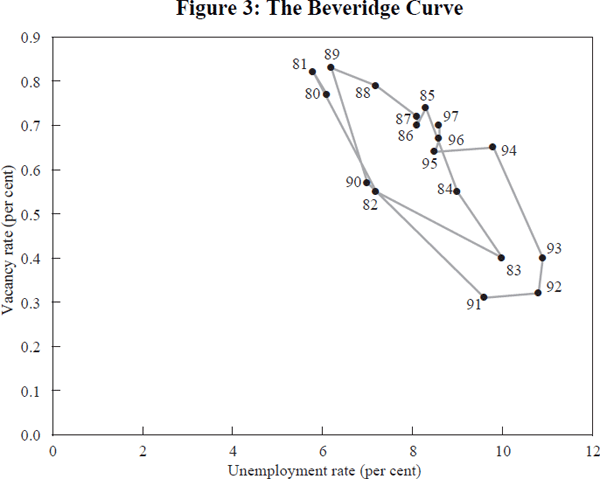
Underpinning the stocks of unemployed people and job vacancies each period are large flows into and out of employment and unemployment. Changes in the efficiency with which the large flows of workers are matched with vacancies shift the position of the Beveridge curve, while over the business cycle the economy shifts along the Beveridge curve.
Using data from 1980 onwards, we estimate the Beveridge curve for Australia in Appendix A. We test to see whether different indicators of the efficiency of process that matches job seekers and job vacancies have led to shifts in the Beveridge curve but find little evidence of this. This suggests that the Beveridge curve has not shifted outwards since the early 1980s, corroborating the evidence from Figure 2 that the natural rate has not changed greatly over the past 15 years. Fahrer and Pease (1993) derive similar conclusions from an analysis of the underlying flows data.
Despite the natural rate remaining relatively constant, the actual unemployment rate has remained above the estimate of the natural rate throughout the 1990s. This may in large part be attributed to the relatively slow decline in inflation expectations (Figure 4). The Phillips curve framework implies that as long as inflation expectations remain above the central bank's inflation target, the economy will operate below potential and unemployment will remain above the natural rate of unemployment. If inflation expectations continue to decline as they have in the past year or two, this would imply that the unemployment rate would converge toward the natural rate.
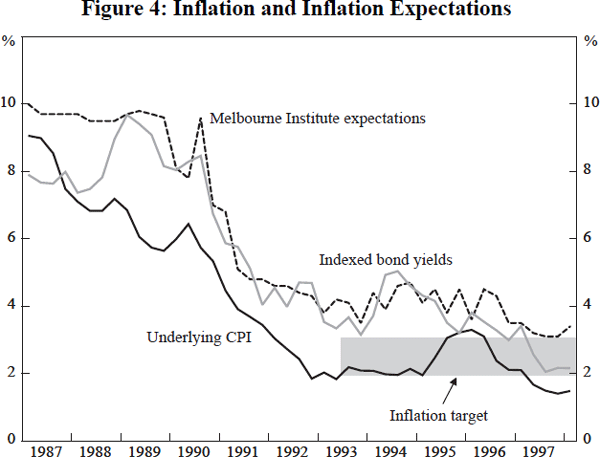
The influence of inflation expectations on the path of the aggregate unemployment rate relative to the natural rate emphasises the need for the central bank to continually ensure that its monetary policy framework is credible and transparent. The more it does so, the faster are inflation expectations likely to fall to the inflation target and the more likely they are to remain anchored at the target in the future.
To some extent, the differences in the estimates of the natural rate shown in Figure 2 reflect the different assumptions about inflation expectations. Crosby and Olekalns (1998) obtain such a high estimate of the natural rate, in large part because they assume that, on average, inflation expectations have equalled actual inflation, thereby implying that the natural rate must, on average, approximate the actual unemployment rate. In contrast, Debelle and Vickery's estimate reflects the fact that inflation has been consistently below their measure of inflation expectations throughout much of the 1990s, so that correspondingly, their estimate of the natural rate has been consistently below the actual unemployment rate.
The OECD (1996) estimate is derived from a wage, rather than a price, Phillips curve, and suffers from the problem that it ignores productivity growth. A trend increase in productivity growth, which is in part reflected in higher wages, will cause the estimate of the natural rate to increase, despite the absence of any inflationary pressure. As there has been an increase in average productivity growth in the 1990s, the OECD measure of the natural rate is above most other measures.
In summary, the aggregate data suggest that there is a strong relationship between movements in real wages and output, and trends in employment and unemployment (we estimate these relationships in Section 4). The large shift upwards in the natural rate of unemployment in the mid 1970s coincided with a rapid increase in labour costs. Subsequently the natural rate has fluctuated around a higher rate of about 7 per cent. In recent years, the actual unemployment rate has remained above estimates of the natural rate, in large part because inflation expectations have only slowly fallen towards the targeted inflation rate.
3. An Aggregate Model of the Labour Market
In this section, we describe a simple framework to examine unemployment from a macroeconomic perspective. Here we present the steadystate version of the model, while later, in Section 5, we add some dynamics to examine the role for monetary policy in assisting the process of labour market adjustment.
In order to address the issue of unemployment, the model requires a departure from the standard neoclassical framework where full employment is generally automatically attained in the long run. Rather, the model is similar to the imperfect competition model of the labour market developed by Layard and Nickell (1986), or the insider/outsider model of Lindbeck and Snower (1988).
Output is produced using two factors, capital (k) and labour (e),[4] so that the economy-wide production function is given by:

In equilibrium, the output supplied by firms equals the output demanded. If not, there is a change in inventory levels. However, we will ignore the role of inventories and assume that output demanded always equals output supplied:

Output will differ from the level of potential output (y*) if either the real interest rate (r), the
instrument of monetary policy, deviates from its equilibrium value  , or through the impact
of fiscal policy (fp). To focus on the role of monetary policy, we
will assume hereafter that fp=0.
, or through the impact
of fiscal policy (fp). To focus on the role of monetary policy, we
will assume hereafter that fp=0.

Turning to the labour market, the number of unemployed people U is definitionally equal to the labour force L less the number of those employed E, so the unemployment rate u can be written as:

The employment demand equation is derived from the cost minimisation decision of the firm (in this case the economy):[5]

where w is the real wage, c is the real user cost of capital, and η denotes the constant-output elasticity of labour demand.[6] This elasticity is the percentage change in employment for a one percentage point change in the real wage, holding output (and the cost of capital) constant. The total effect of a change in the real wage on employment, however, will depend on the extent to which output also changes in response to the change in the real wage (the ‘scale effect’). A fall in the real cost of labour results in the substitution of labour for capital in production. In addition, the firm (economy) is able to move to a higher level of production, thereby employing more labour and more capital.
The existence of the scale effect assumes that there are underutilised resources in the economy. If the unemployment rate is at the frictional unemployment rate (that is, unemployed workers are simply in transition from one employment opportunity to another), then a reduction in the real wage would likely be reversed by the resultant labour market pressure.
The demand for capital is similarly derived from the cost minimisation decision:

The labour supply curve is an aggregate of individuals' labour supply decisions. It depends on the real wage reflecting the labour-leisure choice, and is also assumed to depend on the state of the economy through the encouraged worker effect: when the rate of unemployment is lower, people are more willing to look for work as they expect the probability of finding employment to be greater.

We adopt the simple specification that wages depend on an exogenous component, and the gap between the rate of unemployment and the natural rate of unemployment (u*) which captures the effect of the tightness of the labour market on the level of real wages. This is similar to the wage-setting curve in the Layard-Nickell (1986) model and can be derived from the Shapiro-Stiglitz (1984) model of efficiency wages or the Lindbeck-Snower (1988) model. The exogenous component may reflect the relative bargaining power of insiders and outsiders in the labour market, or the relative bargaining strengths of workers and firms.

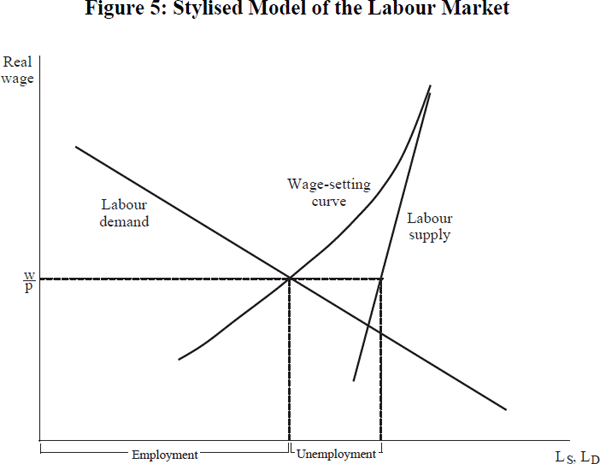
Equations (5), (7) and (8) can be summarised in the following diagram.
Employment is determined by the intersection of the labour demand curve and the wage-setting curve. The level of unemployment is determined by the difference between the level of employment and the size of the labour force at the equilibrium real wage.
To complete the model, we assume that the inflation rate is determined by a standard Phillips curve:

In equilibrium, when interest rates are at their neutral level, output is at potential (y=y*), unemployment is at its natural rate (u=u*) and the real wage is at its exogenous long-run level w′. The above equations imply the following long-run relationships:

Thus, the long-run level of employment is determined by the level of the real wage (and the cost of capital), as is the long-run level of capital stock. This then determines the long-run level of potential output. The long-run level of labour supply, and the natural rate of unemployment are also determined by the exogenous long-run level of the real wage. Monetary policy does not have any impact on either the long-run level of output or unemployment.
We now examine the effects of a fall in the level of the real wage, as a result of a decline in the exogenous component w′. The long-run elasticity of employment with respect to the real wage is:

The numerator of Equation (11) has two components: the first (η) is the own-price elasticity of labour, reflecting the direct increase in labour demand from lower wages. The second is the product of the wage elasticity of capital (ψ), the capital elasticity of output, and the output elasticity of employment (δ): lower wages cause firms to substitute labour for capital, reducing capital demand, which lowers output and consequently reduces employment. Assuming the own price elasticity dominates the employment effects of a lower capital stock, the numerator will be negatively signed overall, thus lower real wages have a net positive effect on employment.
The denominator reflects the scale effect of moving to a higher level of output and is less than one, so the total elasticity is greater than the constant-output elasticity. There is no fall in prices as a result of the decline in the real wage because monetary policy ensures that inflation is maintained at its targeted value.
The elasticity of the unemployment rate with respect to the real wage is:

The first term reflects the direct effect of the change in wages on the unemployment rate, consisting of the net effect of a change in wages on labour supply (σ) and employment. The second term scales this by the size of the encouraged worker effect (κ). The term E*/L*, which equals 1−u*, reflects the fact that the relationship between the unemployment rate and employment and the labour force is in levels but the equations determining employment and the labour force are in logs. This implies that the impact of a real wage change on the natural rate decreases slightly with a lower natural rate.
Also, as mentioned above, there are limits to the impact of a real wage cut on the natural rate. The frictional unemployment rate provides a lower bound for the natural rate. Wage cuts beyond that point would be unwound by wage pressures. In an efficiency-wage framework, firms would not be willing to cut the wage any further in order to maintain the productivity of their workforce. In terms of the insider/outsider model, all members of the labour force would be insiders at this point. In Figure 5, the labour supply curve provides a boundary beyond which the wage-setting curve cannot shift.
A primary aim of the rest of the paper is to quantify the elasticities in Equations (11) and (12) to enable us to estimate the approximate magnitude of the reduction in the rate of unemployment for a given reduction in the level of real wages.
4. Labour Demand and Supply
In this section we estimate the equations for labour demand and supply that form the foundation of the model in Section 3.
4.1 Labour demand
As described in Section 3, the basic form of the labour demand equation that we estimate is derived from the cost minimisation decision of the representative firm (the dual of the profit maximisation decision):

where Equation (5) is extended to take account of a, the level of productivity.
A labour demand curve similar to Equation (5′) can also be derived from an imperfect competition framework, where each firm faces a downward-sloping demand curve for their product, and sets output prices based on expectations of future aggregate prices (Barrell, Pain and Young 1996). Layard and Nickell (1986) derive a somewhat different labour demand equation which controls for the capital stock rather than the level of output. Hamermesh (1993) refers to the Layard-Nickell specification as a short-run labour demand curve in that it captures changes in the demand for labour before the capital stock adjusts. In addition to this theoretical consideration, the problems of measuring the capital stock lead us to adopt a specification that controls for the level of output rather than capital.
Sargent (1978) extends the static labour demand model described in Equation (5′) by assuming that the firm faces costs in adjusting the level of employment, resulting in a partialadjustment specification. Furthermore, the non-stationarity of the variables influencing equilibrium employment suggests that an error-correction framework is the appropriate way to model aggregate labour demand (Amano and Wirjanto 1997), and this is the approach that we adopt.
The core variables of interest in our labour demand equation are employment, real wages, output and the user cost of capital. We do not make an explicit distinction between employment and labour demand in our modelling work, obviating the need to estimate a separate equation linking these two concepts. This contrasts with the labour demand equation in the TRYM model which measures labour demand as the sum of employment and vacancies (Commonwealth Treasury 1996). Since our Beveridge curve regression in Appendix A suggests that the relationship between unemployment and vacancies has not shifted over our sample period, and given that it is difficult to translate vacancies into an hours equivalent, we use actual employment, measured by aggregate hours worked in the non-farm economy, as the dependent variable (hours) in the labour demand equation.
The real wage measure (wage) is total labour costs per hour, which takes into account payroll tax, superannuation contributions and fringe benefits tax in addition to the hourly wage rate, deflated by the non-farm GDP deflator. Output is measured by non-farm GDP(A) and the user cost of capital (ucc) is constructed as a weighted average of the real cost of debt and equity. A full definition of each variable is provided in Appendix B.
We include a linear trend to capture labour productivity. We also tried a measure of multi-factor productivity (MFP), calculated as a weighted average of labour and capital productivity, but found that the variable was generally insignificant and of the wrong sign. In part this may be because MFP is affected by cyclical factors, in particular, the level of capital utilisation. When we included both a time trend and multi-factor productivity in the equation, the time trend was significant whereas MFP was not.
Unit root tests suggest that this set of variables are integrated of order 1, thus warranting the use of an error-correction specification.[7] Hence our initial specification for the labour demand equation is an unrestricted fourth-order autoregressive-distributed-lag (ADL) model expressed in error-correction form:

The βs on the variables in levels are the long-run coefficients while β1 is the speed of adjustment parameter. The rest of the equation where the variables are expressed in first differences represents the short-run dynamics.
The results obtained from estimating Equation (13) are summarised in column 1 of Table 1. Most of the lagged dynamic terms are individually or jointly insignificant. We use the general-to-specific estimation strategy to arrive at a more parsimonious specification which is reported in column 2.
| Sample period | 1978:Q1 – 1997:Q4 | 1978:Q1 – 1997:Q4 | 1978:Q1 – 1997:Q4 | 1969:Q1 – 1997:Q4 | 1979:Q3 – 1997:Q4 | |
|---|---|---|---|---|---|---|
| Estimation method | OLS | OLS | OLS | OLS | IV | |
| (1) | (2) | (3) | (4) | (5) | ||
| Long-run elasticities | ||||||
| Real wage | β2 | −0.42 (0.10) |
−0.42 (0.08) |
−0.40 (0.05) |
−0.68 (0.08) |
−0.39 (0.05) |
| Output | β3 | 1.12 (0.16) |
1.10 (0.12) |
1.09 (0.09) |
1.19 (0.21) |
1.03 (0.10) |
| User cost of capital | β4 | −0.03 (0.19) |
−0.07 (0.16) |
|||
| Time trend | β5 | −0.34 (0.15) |
−0.32 (0.12) |
−0.33 (0.08) |
−0.38 (0.16) |
−0.28 (0.08) |
| Short-run coefficients | ||||||
| ΔHourst1 | γ1 | −0.30# [0.05] |
−0.21 (0.08) |
−0.19 (0.08) |
−0.32 (0.07) |
−0.15 (0.10) |
| ΔReal waget | γ2 | −0.41# [0.00] |
−0.25 (0.06) |
−0.21 (0.05) |
−0.51 (0.10) |
−0.14 (0.11) |
| ΔOutputt | γ3 | 0.24# [0.07] |
0.46 (0.12) |
0.45 (0.11) |
0.10 (0.17) |
0.25 (0.21) |
| ΔUser cost of capitalt | γ4 | −0.47# [0.13] |
−0.16 (0.08) |
|||
| Speed of adjustment | β1 | −0.56 | −0.51 | −0.54 | −0.41 | −0.59 |
| Cointegration test | −3.63 | −6.16*** | −6.70*** | −5.74*** | −5.94*** | |
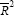 |
0.59 | 0.56 | 0.55 | 0.48 | ||
| LM(1) (p-value) | 0.24 | 0.01 | 0.06 | 0.06 | 0.01 | |
| LM(4) (p-value) | 0.32 | 0.01 | 0.13 | 0.21 | 0.01 | |
| Notes: The dependent variable is the change in aggregate hours worked. All variables in logs except the user cost of capital. Estimation by ordinary least squares (columns 1 to 4), and instrumental variables (column 5). Standard errors in parentheses except: # for the ADL(4) model in column 1, the reported short-run coefficients are the sum of the short-run coefficients for each variable. The value in square brackets is the p-value for the F-test that the short-run coefficients are jointly significant. *, ** and *** refer to significance at the 10 per cent, 5 per cent and 1 per cent levels of significance for the Kremers, Ericsson and Dolado (1992) test for cointegration. The LM test is the Breusch (1978) test for first and fourth order autocorrelation. | ||||||
The user cost of capital was insignificant and had the wrong (negative) sign, so in the third column we present our preferred specification which excludes the user cost of capital. The exclusion of the user cost of capital also reduces the problem of autocorrelation.
Because of the potential endogeneity of the contemporaneous values of wages and output in the labour demand equation, we estimated it using both instrumental variables and ordinary least squares. Initially we estimated the equation using two-stage least squares where the instruments were the exogenous variables from a system which included an equation for output and the wage in addition to the labour demand and supply equations. We did not estimate the output equation or the wage equation directly but only used them to provide instruments: namely US GDP for output (Gruen and Shuetrim 1994), and award wages and a measure of union power for wages.
We tested the over-identification restrictions implied by our instrument set and found that they were rejected.[8] Consequently we also estimated the equations using a smaller instrument set, which excluded the contemporaneous values of the instruments. The results of this estimation are reported in column 5 of Table 1. A Hausman test failed to reject the null hypothesis of exogeneity using either instrument set,[9] so we focus on the OLS estimates in the remainder of the discussion. As column 5 indicates, the results are little changed if IV is used. Quandt and Rosen (1989) also found that endogenising output made little difference to coefficient estimates in an aggregate labour demand curve for the United States, and that exogeneity of output could not be rejected statistically.
We estimate the equation over two time horizons. Columns 1, 2, 3 and 5 use data from 1978:Q1 to 1997:Q4 where labour demand is measured by the aggregate hours worked in the non-farm economy. As this series is not available before 1978, column 4 uses data from 1969:Q1 to 1997:Q4, measuring labour demand by aggregate hours worked in the whole economy adjusted for the share of non-farm output in total output. Hours worked in the non-farm economy is less volatile than that in the whole economy thus accounting, in part, for the lower explanatory power in column 4.
Our estimate of the long-run real wage elasticity of employment of −0.4 is smaller than the previous estimates of around −0.8 (Lewis and Kirby 1988; Pissarides 1991; Russell and Tease 1991). Our estimates lie inside the range of −0.15 to −0.75 that Hamermesh (1993) reports in his survey of the international labour demand literature as ‘a reasonable confidence interval’ for the real wage elasticity.
The difference between our estimates and the other Australian studies appears to be partially due to the sample period used, and partially a result of different model specifications.[10] When we estimate the model over the longer period (column 4), we find a significantly higher wage elasticity of −0.67. To examine how the wage elasticity has evolved over time, we estimated rolling regressions of the labour demand equation over fifteen-year windows. This indicates that the wage elasticity has declined (in absolute value) since the mid 1980s but has been relatively constant over the 1990s at around −0.4. Furthermore, Russell and Tease use real unit labour costs as their measure of labour costs. In the appendix to their paper they report a specification that uses a measure of real wages which yields a significantly lower wage elasticity of −0.36.
Our estimate of the long-run output elasticity is, however, consistent with previous estimates. The output elasticity is not significantly different from one in either the shorter or longer sample, implying that a one per cent increase in output leads to a one per cent increase in employment. The TRYM model actually imposes a unitary elasticity rather than estimates it.
The speed of adjustment to long-run equilibrium is generally invariant to the specification used. The estimate of around 0.5 implies that 95 per cent of the adjustment back towards long-run equilibrium is completed within four quarters after a shock. The specifications in Table 1 all assume that the speed of adjustment is constant. We also estimated a specification of the labour demand curve where the speed of adjustment depends on the tightness of the labour market and hiring and firing costs (Burgess 1993). However, the evidence suggested that the speed of adjustment is invariant to the state of the business cycle.
In summary, our preferred specification for labour demand contains only real labour costs, output, and a linear trend. The constantoutput real wage elasticity appears to be around −0.4, while the output elasticity is around 1.
4.2 Labour supply
The labour supply or participation rate equation can be derived from an aggregate version of the individual's labour/leisure choice, in which labour supply is determined by the wage, the prices of goods in an individual's consumption basket, and non-wage income. An individual will supply labour, provided that the payoff from accepting employment exceeds their reservation wage.
We measure the consumption wage (cwage) using after-tax average weekly earnings deflated by the consumer price index, and also include in the specification the real level of unemployment benefits (ben) which is likely to have a major influence on the reservation wage.
In the basic labour supply model, the real consumption wage represents the payoff for the individual supplying labour to the market. However, if there is uncertainty about the prospects of obtaining employment, the probability of finding work will also affect the payoff. Consequently, the participation rate tends to be pro-cyclical as a result of this ‘discouraged/encouraged’ worker effect.
On the other hand, viewing the labour supply decision from the household level, a major component of an individual's non-wage income is the income of their spouse. If their spouse loses their job as the economy enters a downturn, an individual may enter the labour force to offset the decline in household income. This ‘added’ worker effect will dampen the discouraged worker effect. However, Bradbury (1995) finds little evidence for a significant added worker effect using Australian Department of Social Security data.
A review of early Australian models of aggregate labour supply is provided in Dunlop, Healy and McMahon (1984). They find that in many of the studies surveyed, key parameter estimates were unstable over time, and that the model specifications used were not linked closely enough to economic theory. Since that time, much of the macroeconomic research on labour force participation has focused on separating trend and cyclical influences on labour supply (Dixon 1996; Dowrick 1988; Gregory 1991), rather than modelling labour supply decisions in terms of behavioural variables.
Most of the relevant existing literature on participation rates uses the employment to population ratio to capture the discouraged worker effect (e.g. Dowrick 1988; Elmeskov and Pichelmann 1993; Pissarides 1991; Stacey and Downes 1995). However, there is a definitional link between the employment to population ratio and the participation rate:
Labour Force/Population = Employed/Population + Unemployed/Population
Any divergence between the two occurs only because of changes in the unemployment rate. Consequently, the direction of causality between the two variables is unclear. Elmeskov and Pichelman suggest that the main linkage runs from employment to participation, although they acknowledge that the causality is most probably bi-directional. If an exogenous increase in labour supply increases employment by putting downward pressure on wages, then the employment to population variable is rendered endogenous in a participation rate equation.
The critical factor in the labour supply decision is the expected probability of finding employment. Therefore we measure the discouraged worker effect using the vacancy rate (vac), or the vacancy rate relative to the pool of unemployed (vacu).
We estimate separate equations for male and female participation rates, reflecting their divergent behaviour over the sample period. The lower participation rates of women and the higher proportion of women in part-time employment has historically been indicative of a more marginal attachment to the labour force. Thus, we might expect their participation to be more sensitive to the discouraged worker effect. For married women with children, child care costs may also influence their labour supply decision, so we include the ratio of child care costs to female average weekly earnings (child) in the female participation rate equation. We also test for the impact of housing loan affordability (home) on female labour supply as suggested by Connolly and Spence (1996).
A major change which has influenced the supply decision of both genders over the sample period has been the increase in school retention rates and the increased participation in tertiary education. We control for this effect using the proportion of men and women in full-time education (edu).
However, some part of the trends in male and female participation may reflect changes in social preferences which we are unable to model directly, so we also include a time trend in both specifications. Thus our general specification for the participation rate equation for each gender is given by:
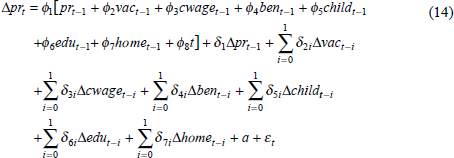
The expression in the square brackets describes the long-run relationship, with φ1 the speed of adjustment parameter while the short-run dynamics are described in the rest of the equation.[11]
The model is estimated using quarterly data from 1979:Q3 to 1997:Q4. As in the labour demand equation, we initially used instrumental variables estimation to allow for the possibility of endogeneity of the vacancy rate and the wage. However, again a Hausman test failed to reject the hypothesis of exogeneity,[12] so in Table 2, we report the results from estimating the equation using ordinary least squares. The lagged dynamic terms were insignificant so the first column for each gender reports the results with only contemporaneous dynamic terms.
| Male participation rate | Female participation rate | |||||||
|---|---|---|---|---|---|---|---|---|
| (1) | (2) | (3) | (4) | (5) | (6) | |||
| Long-run elasticities | ||||||||
| Vacancy rate | φ2 | 0.54 (0.79) |
1.48 (0.68) |
4.45 (2.65) |
6.22 (1.84) |
|||
| Vacancy rate/ unemployment rate |
φ2 | 9.04 (2.80) |
28.87 (6.18) |
|||||
| Real wage | φ3 | −3.80 (2.80) |
−6.84 (8.85) |
|||||
| Education | φ6 | −0.74 (0.28) |
−0.57 (0.30) |
−0.49 (0.25) |
2.89 (0.97) |
3.40 (0.88) |
2.95 (0.61) |
|
| Time trend | φ8 | −6.00 (0.43) |
−5.90 (0.50) |
−5.72 (0.43) |
10.21 (2.67) |
8.62 (2.24) |
10.60 (1.55) |
|
| Short-run coefficients | ||||||||
| ΔVacanciest | δ2 | −1.05 (0.39) |
−0.83 (0.36) |
−0.61 (0.55) |
−0.51 (0.49) |
|||
| ΔVacancy rate/ unemployment ratet |
δ2 | −3.87 (1.99) |
−0.98 (2.78) |
|||||
| ΔReal wagest | δ3 | −0.41 (0.92) |
−0.40 (1.33) |
|||||
| ΔEducationt | δ6 | −0.17 (0.28) |
0.11 (0.49) |
|||||
| Speed of adjustment | φ1 | −0.29 | −0.25 | −0.30 | −0.17 | −0.15 | −0.19 | |
| Cointegration test | −3.93* | −3.83** | −4.52*** | −3.19 | −3.47 | −3.99** | ||
 |
0.22 | 0.23 | 0.25 | 0.22 | 0.25 | 0.23 | ||
| LM(1) (p-value) | 0.70 | 0.77 | 0.87 | 0.37 | 0.50 | 0.33 | ||
| LM(4) (p-value) | 0.57 | 0.59 | 0.47 | 0.07 | 0.05 | 0.09 | ||
| Notes: The dependent variable is the change in the male participation rate (columns 1 to 3) or the female participation rate (columns 4 to 6). Standard errors in parentheses. Estimation by ordinary least squares. *, ** and *** refer to significance at the 10 per cent, 5 per cent and 1 per cent levels of significance for the Kremers, Ericsson and Dolado (1992) test for cointegration. The LM test is the Breusch (1978) test for first and fourth order autocorrelation. | ||||||||
We found that the cost of child care and the real unemployment benefit were insignificant in both the male and female participation rate equations. In contrast to Connolly and Spence (1996), we found that home loan affordability was not an important influence on the labour force participation decisions of women, although it had the expected sign: increased home loan affordability causes women to leave the labour force. One possible explanation for our result is that greater home loan affordability mainly results in families purchasing more expensive homes, rather than reducing their repayment burdens.
For both genders, wages were found to be statistically insignificant, and were also negatively signed, suggesting that the income effect of a change in wages at least offsets the substitution effect. Lewis and Kirby (1988) find a significant positive real wage elasticity, although inferences based on their standard errors must be qualified, since they estimate an equation in levels with trending variables. Furthermore, their labour supply equation has a fundamentally different specification to ours, since their dependent variable is the quantity of labour supplied, but they do not control for the size of the working-age population. In contrast, since our dependent variable is the participation rate, we in effect impose linear homogeneity between population size and the level of labour supply.
Our results are consistent with the large international body of microeconomic research on labour supply, which generally finds only a weak, and often tenuous, relationship between wages and hours of labour supplied,[13] and it is also consistent with the aggregate participation rate equation in Pissarides (1991). Therefore in the remaining columns, we exclude wages from the specification.
The evidence for a cointegrating relationship is weaker than in the labour demand equation, where the null of no cointegration was clearly rejected.[14] The speed of adjustment towards equilibrium is relatively slow: for the specifications in columns 2 and 5, 25 per cent and 15 per cent respectively of the gap between actual and equilibrium labour force participation is closed each quarter.
The results suggest that the encouraged worker effect is an important influence on labour force participation and the economic magnitude is quite large. We also find that the size of the encouraged worker effect is much larger for women than for men, confirming the view of Gregory, McMahon and Whittingham (1985) that many non-participating females represent a latent stock of labour supply that would enter the labour force in the presence of favourable labour market conditions. Between 1991 and 1997, the vacancy rate increased by approximately 0.6 (from 0.3 per cent to 0.9 per cent); based on the models in columns 2 and 5, an increase of this magnitude would raise the female participation rate by 3.7 percentage points, and the male participation rate by 0.9 percentage points. The results are broadly similar whether we use either vacancies or the ratio of vacancies to unemployment to measure the encouraged worker effect.
Participation in full-time education also appears to have an influence on labour force participation. In the male participation rate model (column 2), education is negatively signed (although not significant), reflecting the fact that for men, education is a substitute for time which would otherwise have been spent in the labour market.[15] In the female model, however, the coefficient on education is positively signed and significant. One explanation of this finding is that education is correlated with unobservable demographic change variables which are driving the persistent upward trend in the female participation rate. Another explanation is that in contrast to males, education provides an opportunity for females to gain skills which will allow them to gain employment.
The time trend variable is highly significant in both the male and female participation rate equations, suggesting that demographic and attitudinal changes, which we were unable to capture explicitly within our modelling framework, have been an important influence on the observed changes in participation rates.
In conclusion, our preferred specification for labour supply includes the encouraged worker effect (measured by the vacancy rate), participation in education and a time trend.
5. Reducing Unemployment
In this section, we use the model presented in Section 3, and the parameters of the model estimated in Section 4 to provide some benchmark estimates for the size of the reduction in the level of real wages needed to achieve a permanent reduction in the rate of unemployment. That is, we quantify the expression for the elasticity of unemployment with respect to the real wage derived in Equation (12). We also examine the adjustment of the economy to the lower unemployment rate, and the role that monetary policy can play in that adjustment.
The model is somewhat stylised but it highlights the main relationships involved. Later in the section, we compare the results obtained with those from two more fully specified macroeconomic models of the Australian economy.
We assume that initially the economy is in equilibrium at the current natural rate of unemployment determined by the equilibrium real wage, itself a function of the existing set of labour market institutions. The research reported in Section 2 suggests that the natural rate is currently likely to be somewhere between 7 and 7.5 per cent. Over time, the unemployment rate should gradually fall to this level without any change in the exogenous component of the real wage or labour market institutions.
The basic core of the model consists of the labour demand and labour supply equations. The parameters in the labour demand curve are taken from column 3 of Table 1, where the real wage elasticity is −0.4 and the output elasticity is 1.09. For the purposes of the simulation we assume that productivity remains constant. Equivalently, output and productivity could grow at a constant rate with no net effect on employment.
We make two different assumptions about the process for output. Firstly, we assume that output is invariant to changes in employment; that is, the scale effect is one. This is likely to provide a lower bound on the impact of the wage change. Quandt and Rosen (1989) however, provide evidence that the scale effect is one in the United States.
Secondly, we assume that output increases proportionately to labour's share of income, 0.58. That is, a one percentage point increase in employment leads to a 0.58 percentage point increase in output, which amplifies the own price elasticity of employment by 2.4 (=1/(1–0.58)). That is, the scale effect is 2.4. This is similar to the longrun response of output relative to employment in the TRYM model in response to a permanent decline in the natural rate.[16]
We use the participation rate equations for males and females in columns 2 and 5 in Table 2.[17] These are weighted together to give an aggregate participation rate equation by the share of males and females in the working-age population as at December 1997 (0.49 and 0.51 respectively). We assume that the participation rate in education remains constant at its December 1997 level. We also assume that there are no further trend changes in participation rates. To the extent that the aggregate participation rate is still rising through time, the necessary decline in the real wage to achieve a particular unemployment rate would be larger.
The encouraged worker effect is measured using the vacancy rate, so to relate this to movements in the unemployment rate, we use the Beveridge curve estimated in Appendix A. The employment demand curve is estimated in terms of hours while the Beveridge curve and participation rate equations are estimated in terms of people. To map one into the other, we assume that average hours worked remain at their level of end 1997.
Starting from an unemployment rate of 7.5 per cent, we consider the impact of a reduction in the real wage of two percentage points. The reduction is achieved by a decline in the exogenous component in the wage equation. The exact mechanism by which this could be achieved is beyond the scope of this paper, but could include reforms that permanently changed the balance between insiders and outsiders in wage-setting. It does not imply that only real wages at the lower end of the wage distribution are reduced, but rather that the average real wage in the economy is lowered. Furthermore, given an inflation target of 2 to 3 per cent, this implies that nominal wages do not have to fall to achieve the cut in real wages. Thus issues of nominal wage rigidity can be ignored.
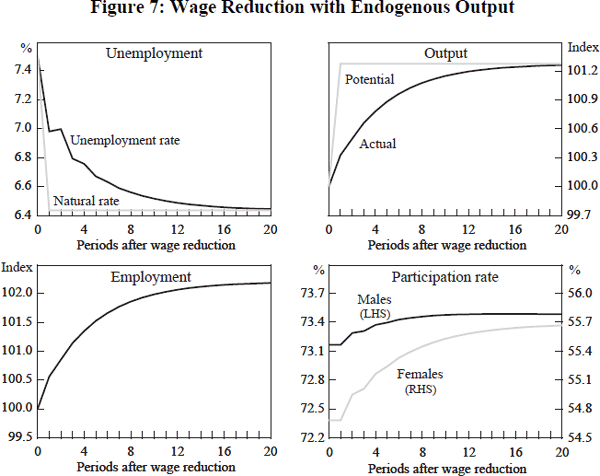
We analyse the sensitivity of these results to different assumptions about the model parameters, including in particular, the effect of monetary policy on the outcomes. First of all, we assume that monetary policy holds the real interest rate at its neutral value throughout. Given this assumption, Figure 6 shows the impact on unemployment, employment and male and female participation rates of the two percentage point reduction in the real wage when the scale effect is 1. Figure 7 shows the results when the scale effect is 2.4.

When the scale effect is 1, there is a fall in the natural rate of unemployment of 0.41 percentage points. The adjustment of unemployment to the new lower natural rate is rapid, given the absence of much dynamics in the labour demand and supply equations. The different patterns of adjustment of the male and female participation rates reflect the different sizes of the encouraged worker effects, and the different speed-of-adjustment parameters. Employment adjusts by a greater proportion (0.8 per cent) than the fall in unemployment, because the encouraged worker effect dampens the impact on unemployment of the fall in the real wage: every extra 1 per cent of employment induces a 0.3 percentage point increase in the participation rate, so that the net impact on unemployment is only 0.5 percentage points.
When we allow for the scale effect, the 2 per cent decline in the real wage leads to a fall in the natural rate of unemployment of 1.1 percentage points, and a 2.2 per cent rise in employment. The adjustment to equilibrium is somewhat slower than the previous scenario, reflecting the fact that the scale effect operates with a lag through the error-correction mechanism in the employment equation. Nevertheless, much of the fall in unemployment is complete within a year of the fall in the wage.
Thus far, we have assumed that monetary policy has maintained a neutral stance throughout the adjustment process. We now examine the role that monetary policy can play in assisting the adjustment to the lower unemployment rate.
We assume that monetary policy affects output with a one quarter lag. In reality, the lags of monetary policy are longer than this (Gruen, Romalis and Chandra 1997), but we have adopted this assumption to simplify the argument. Monetary policy is determined optimally in a forward-looking manner to minimise deviations of output from potential and inflation from its targeted value:
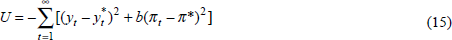
Monetary policy cannot affect the long-run level of the natural rate or potential output. Attempts to stimulate the economy above potential will only result in inflation. However, it minimises both the duration and the size of divergences of output from potential, and hence unemployment from the natural rate.
Inflation is determined by a Phillips curve (Equation (9)) where the coefficient β on the output gap is 0.1. For simplicity, inflation expectations are assumed to remain constant at the targeted inflation rate,[18] implying that the deviation of inflation from target is directly proportional to the output gap. Given this, the monetary policy rule implies that real interest rates are adjusted in proportion to the expected output gap.
The above exercise assumes that the central bank realises the decline in the real wage is permanent and understands the full extent of its impact, which may not be very realistic. If the central bank can only infer the structural change in the economy from observed variables, it might initially perceive the rise in output shown in Figure 7 as being inflationary and will act to counteract it. Over time, inflation will, in fact, fall as a consequence of the output gap that has thereby been generated. So only gradually would the central bank be able to realise that output is rising only in line with potential, and is not, in fact, inflationary. The inflation-targeting framework ensures that the economy eventually returns to potential as the central bank reacts to the resultant disinflation, but in the interim, unemployment will be higher than otherwise. If instead of an inflation target, the central bank targets the output gap, the inflation rate will continue to fall, but the central bank will still attempt to counteract the inflationary impulse of the perceived positive output gap. We illustrate this point in Figure 8.
We assume that the central bank only gradually learns about the increase in potential, as a result of the structural change in the labour market. Let y*CBt be the central bank's estimate of potential output at time t, and y*At be the actual level of potential output. Assume that the central bank adjusts its estimate of potential output in the following manner:

This is equivalent to the central bank placing some weight on the wage change being temporary and some weight on it being permanent.
Figure 8 shows the paths of output and unemployment for ω = 0.1, 0.3 and 1.
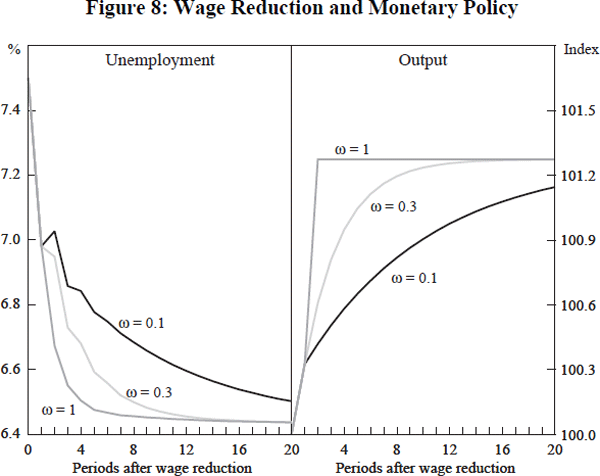
When ω = 1, the central bank immediately realises the wage change is permanent. Interest rates are set so that output rises to its new long-run level within one quarter: an output gap persists only as long as the lag in the effect of monetary policy. Unemployment does not immediately attain its long-run value, given the dynamics of the participation rate, but the gap between the natural rate and the actual rate of unemployment is less than that of neutral policy. Thus, monetary policy can increase the speed with which the unemployment rate falls to the new natural rate during the adjustment phase provided it recognises that the wage change is permanent.
In practice, it will be difficult for the central bank firstly to identify the change, and secondly to ascertain whether it is permanent or not. We now take account of this fact. When ω is set equal to 0.1, the central bank believes that the chance that the wage change is permanent is quite small. When the central bank sees stronger output growth, it believes that output has risen above potential and acts to counteract that with higher interest rates, although this is mitigated to some extent by the fact that inflation is falling below its targeted level because, in reality, output is below potential. Unemployment clearly takes a longer time to reach the lower steady state level. The gap between the actual unemployment rate and the natural rate of unemployment is greater than 0.1 percentage points for over three years. In the intermediate case when ω = 0.3, the unemployment rate still falls more slowly than if the central bank believed the wage change was permanent immediately.
Above we have assumed that the real wage has fallen but the central bank believes it is temporary. If on the other hand, the central bank believes that the wage change is permanent when in fact it is temporary, it will believe that the level of potential output has risen (when in fact it has not) and will therefore loosen policy. This will lead to a rise in inflation, which will need to be counteracted by a period of unemployment above the natural rate.
There are clearly problems of possible misinterpretation in both directions. In practice, it is extremely difficult to identify whether such developments in the labour market are temporary or permanent, particularly in a period of ongoing structural change. Nevertheless, the results suggest that the central bank can enhance the adjustment process by constantly monitoring and assessing the economy in light of new evidence about developments in the labour market. An inflation target can assist this process because the focus on the inflation rate will alert the central bank to the possibility of structural change when the inflation rate persistently undershoots its forecast value.
Thus far, we have ignored the effect of changes in the capital stock as a result of the change in the real wage, and the induced increase in employment. The fall in the real wage will cause firms to substitute labour for capital. However, the scale effect will induce more investment and an expansion of the capital stock. This process is difficult to quantify in the framework used here, however, the Murphy and TRYM models explicitly address this issue (Commonwealth Treasury 1996; Murphy 1992). Both models have a direct link between the natural rate and the real wage, and have a labour market structure very similar to the one used here. In terms of the model in Section 3, the primary difference is that these models incorporate a direct feedback from the capital stock to the real wage in the medium to long term.
Brooker (1993) examines the effect of a cut in the real wage in both models and finds that the fall in the real wage initially results in a decline in the capital stock as firms substitute towards labour. However, in the longer term the reduction in the real wage increases the expected return on capital and hence leads to an increase in investment, which in turn leads to a further increase in employment. This process is eventually curtailed by a feedback mechanism from the increased employment to a higher real wage. In the long run, the real wage can even rise above its initial value, but the initial decline is still necessary to stimulate the adjustment. There is still the need for a permanent shift in labour market institutions. The new long-run equilibrium with the higher real wage and capital stock is not compatible with the initial institutional framework.
The two models tend to generate a slightly higher increase in employment as a result of a decline in the real wage than that presented here, because of the long-run increase in the capital stock. The TRYM model also finds that the lower unemployment rate decreases the amount of unemployment benefits paid. This then permits the government to reduce the tax rate resulting in a rise in after-tax wages which can offset the initial decrease (Stacey and Downes 1995).
In light of these caveats, we examine the sensitivity of the results to different parameter values. Table 3 shows the effect on the long-run change in the unemployment rate as a result of the 2 per cent reduction in the real wage, of varying the real wage elasticity parameter, and the scale effect parameter. We allow the wage elasticity to be as high as −0.8 consistent with that in other Australian studies of labour demand (see Section 4.1). We let the scale effect take the values of 1 (consistent with the US evidence), 2.4 (corresponding to labour's share of income of 0.58) and an intermediate value of 1.33. The table shows that doubling the wage elasticity leads to around twice as large an impact on the unemployment rate. As the scale effect increases, the decline in unemployment is larger, although not proportionately so.
| Wage elasticity | Scale effect | ||
|---|---|---|---|
| 1 | 1.33 | 2.4 | |
| −0.40 | −0.41 | −0.56 | −1.07 |
| −0.68 | −0.68 | −0.92 | −1.71 |
| −0.80 | −0.80 | −1.07 | −1.97 |
Finally, we use the model to examine the impact of the 1970s increase in the real wage on the natural rate. The natural rate in 1973 before the large increase in wages was around 4.4 per cent. From 1973 to 1975, real unit labour costs increased by 6.1 per cent. In the framework we have estimated here, this would lead to an increase in the natural rate of just under 3 percentage points to around 7.3 per cent. This is of a similar order of magnitude to the shift in the natural rate in the TRYM model in the mid 1970s.
The increase in labour supply in the 1970s required a transitory decline in the real wage to enable the necessary investment to occur so that the additional labour could be employed. In fact, the reverse occurred and the real wage rose. This rise is likely to have discouraged investment and led to a sub-optimal level of the capital stock, the effects of which have persisted, so that, despite the real wage falling to the levels of the early 1970s, the unemployment rate has remained well above those levels. A larger capital stock would have allowed more labour to be employed at the existing real wage, by increasing the productivity of the workforce.
6. Conclusion
The primary conclusion of this paper is that the average level of real wages can have a significant impact on the long-run level of unemployment in the economy. The paper has suggested that slower growth in real wages of 2 per cent for a year could lead to a permanent reduction in the unemployment rate of around 1 percentage point. The final decrease in the unemployment rate may indeed be even larger if there is a long-run increase in investment and the capital stock as a result of the fall in wage growth.
Estimates of the long-run or natural rate of unemployment suggest that it rose sharply in the 1970s associated with the rapid rise in labour costs at the time but has since fluctuated between 6 and 7.5 per cent. Our estimates imply that the wage rise in the 1970s may have increased the natural rate by around 3 percentage points.
Results from our labour demand equation confirm the findings of previous studies that the elasticity of employment with respect to output is close to unity. Our estimate for the wage elasticity of employment of −0.4 is lower than empirical work from the 1980s, but is consistent with a range of international evidence and the estimates reported in Dungey and Pitchford (1998) in this volume. We find clear evidence that labour supply is sensitive to the state of the labour market – the ‘encouraged worker effect’. The size of this effect is much larger for women than for men. We also find that labour supply is relatively invariant to real wages.
Monetary policy does not have any impact on the natural rate but can seek to ensure that the unemployment rate remains as close as possible to the natural rate by avoiding, as far as possible, sharp swings in the business cycle. Maintaining a relatively constant rate of economic growth can reduce the average unemployment rate, although the natural rate provides a lower bound. Inflation expectations also play a critical role. The fact that the unemployment rate has been above the natural rate for most of the 1990s, is in part due to the slow adjustment of inflation expectations to the lower inflation rate.
Monetary policy can also play a role in the transition process to a lower unemployment rate in the event of structural change in the labour market. In practice, it is extremely difficult to identify whether developments in the labour market are temporary or permanent. However, forward-looking monetary policy that is cognisant of the structural change going on in the economy can reduce the amount of excess unemployment during the adjustment phase. An inflation-targeting framework for monetary policy is beneficial in this regard by ensuring that monetary policy is forward-looking and because developments in the labour market are a crucial component of the outlook for inflation.
Appendix A: The Beveridge Curve
In this Appendix, we estimate a Beveridge curve for Australia and examine whether changes in the efficiency with which workers are matched with the vacant jobs have caused the curve to shift over time, in order to indirectly assess whether those factors are likely to have caused the natural rate to rise over time.
We measure changes in the efficiency of the matching function by: an index of sectoral dispersion to capture mismatch between skills supplied and demanded (Lilien 1982); the standard deviation of state unemployment rates to measure geographic mismatch; the share of young people in the labour force, as they tend to have a greater proclivity to move from job to job to find one that suits; and the real level of the unemployment benefit which may affect search intensity.
We estimate the curve using an error-correction specification over the period 1979:Q3–1997:Q4. We find that none of the above characteristics of the efficiency of the labour market are statistically significant. Furthermore, a time trend to capture any ongoing shift in the curve caused by other factors was also insignificant, indicating that the Beveridge curve has not shifted over the period. However, Figure 2 suggests that most of the rise in the natural rate may have occurred in the mid 1970s, which predates the sample period employed here (Harper 1980).
In Table A1, we report our estimate of the Beveridge curve which we use in Section 5. The results confirm the negative relationship between unemployment u and vacancies v suggested by Figure 3.
| Δut = 0.16 − 0.20 Δvt + 0.27 Δut−1 − 0.09(ut−1 − 0.79vt−1) (0.05) (0.04) (0.10) | ||
|---|---|---|
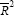 = 0.65 = 0.65 |
LM(1) p-value = 0.21 | LM(4) p-value = 0.38 |
| Notes: The Kremers, Ericsson and Dolado (1992) test for cointegration has a test statistic of −3.06, which lies in the indeterminate region between the normal and Dickey-Fuller distributions. Standard errors are in parentheses. | ||
Appendix B: Data Sources
| Data | Source |
|---|---|
| Unemployment rate | ABS cat. no. 6203.0, Table 1. |
| Employment to population ratio | Ratio of total employment to civilian population aged 15 and over. ABS cat. no. 6203.0, Table 1. |
| Inflation expectations based on indexed bond yields | Nominal 10-year bond yield, deflated by yield on a 10-year capital indexed bond (Reserve Bank Bulletin Table F.2). |
| Melbourne Institute inflation expectations | Median inflation expectations. Melbourne Institute Survey of Consumer Inflationary Expectations, Melbourne University. |
| Real labour costs per hour worked | Australian Treasury, <http://www.treasury.gov.au>. |
| Real unit labour costs | Australian Treasury, <http://www.treasury.gov.au>. |
| Underlying consumer price index | ABS cat. no. 6401.0, Table 10. |
| Real child care costs | Nominal index of child care costs (ABS cat. no. 6401.0, Table 6), deflated by the CPI (ABS cat. no. 6401.0, Table 1). |
| Average weekly earnings | ABS cat. no. 5206.0, Table 1, deflated by the final consumption deflator (ABS cat. no. 6401.0, Table 1). |
| Non-farm GDP(A) | ABS cat. no. 5206.0, Table 54. |
| Real user cost of capital | Weighted average cost of capital, based on the tax-adjusted cost of debt, derived from the business indicator rate (RBA Bulletin, Table F.3) and the real cost of equity, calculated using a Gordon dividend growth model. |
| Total factor productivity | Non-farm index. ABS cat. no. 5234.0, Appendix 1. |
| US GNP | Datastream, USGDP… D. |
| Award wages | ABS cat. no. 6312.0, Table 1, deflated by the CPI. (ABS cat. no. 6401.0, Table 1). |
| Union coverage | ABS cat. no. 6323.0, Table 3. |
| Male (female) real consumption wage | Male (female) average weekly earnings per hour worked multiplied by (1 – implied personal tax rate). Real AWE is from ABS cat. no. 6302.0, Table 2, deflated by the CPI (ABS cat. no. 6401.0, Table 1). Average hours worked by gender is from ABS cat. no. 6203.0, Table 17. The implied personal tax rate is calculated as the ratio of net tax paid by individuals (NIF database) to wages, salaries and supplements (ABS cat. no. 5206.0, Table 22). |
| Real unemployment benefits | Nominal unemployment benefit for a single adult deflated by the CPI (ABS cat. no. 6401.0, Table 1). |
| Vacancy rate | ABS cat. no. 6354.0, Table 1. |
| Home loan affordability | Ratio of average household disposable income to initial payment on average sized owner-occupier home loan. |
| Male (female) participation in full-time education | Proportion of 15–24 year olds participating in full-time education (ABS cat. no. 6227.0). Interpolated to give a quarterly time series. |
Footnotes
We thank Philip Lowe, Peter Downes, Jeff Borland, Mardi Dungey, John Pitchford and colleagues at the Reserve Bank for helpful discussions. [*]
At the time, there was a vigorous debate about the impact of these wage movements on employment – the ‘real wage overhang’ debate. See Chapman (1990), Gregory and Duncan (1979) and Indecs (1986) for a summary of the debate. [1]
A similar debate has occurred on the role of the Accord in the observed wage outcomes over this time. Cockerell and Russell (1995) and Pissarides (1991) find no impact of the Accord on wages once other influences are accounted for, whereas Chapman and Gruen (1990) and Watts and Mitchell (1990) conclude the opposite. [2]
Reliable vacancies data are not available prior to 1979. However, it appears likely that the Beveridge curve shifted outwards during the 1970s (Harper 1980). [3]
Lower-case letters denote logs, upper-case letters, levels. [4]
For a full treatment see Hamermesh (1993). [5]
η is not necessarily the firm-level elasticity of labour demand because of issues of aggregation (Hamermesh 1993, p. 64). Thus the estimates of η obtained in Section 4 below are not directly comparable with those obtained from microeconomic studies. [6]
We use the Dickey-Fuller T(ρ-1) statistic to test for the order of integration of each of the variables. Each series was found to be I(1) based on a 5 per cent level of significance. [7]
Using the Hausman (1983) test for over-identification, the value of the χ2(11) test statistic was 29.54. [8]
The value of the χ2(8) test statistic was 2.76. [9]
The differences are not due to the measure of wages that we use. A similar long-run elasticity was obtained using a number of different wage measures. [10]
The male and female participation rates, and each of the right-hand side variables, were found to be I(1) at the 5 per cent level of significance, based on the Dickey-Fuller T(ρ-1) test. [11]
The value of the Hausman χ2(9) test statistic was 1.88 for the male participation rate equation, and χ2(9) = 2.73 for the female participation rate equation. [12]
Killingsworth (1983), and the papers by Pencavel (1986) and Killingsworth and Heckman (1986) in the Handbook of Labor Economics, provide a broad survey of this literature. Taken as a whole, this body of work suggests that the wage elasticity of labour supply is negligible for men, and is perhaps slightly positive for women. The small body of Australian research on female labour supply has, however, generally found quite high positive real wage elasticities. This literature is surveyed in Kenyon and Wooden (1995). [13]
While for males the null hypothesis of no cointegration is rejected, for females, the test statistic lies in the ‘unclear region’, between the normal and Dickey-Fuller critical values. [14]
While people still participate in the labour force while in the education, the participation rate is lower than that for the rest of the population. [15]
See Commonwealth Treasury (1996), pp. 34–37. There it is assumed that monetary policy does not accommodate the increase in real activity but rather lets the price level fall. [16]
We ignore the dynamic terms in the two labour supply equations which tended to generate some instability in the simulations. This only affects the adjustment process, not the final result. [17]
This implies that expectations are not rational. Adding a process for inflation expectations would only serve to further complicate the dynamics. [18]
References
Amano, R. and T. Wirjanto (1997), ‘An Empirical Study of Dynamic Labour Demand with Integrated Forcing Processes’, Journal of Macroeconomics, 19(4), pp. 697–715.
Barrell, R., N. Pain and G. Young (1996), ‘A Cross-country Examination of the Demand for Labour in Europe’, Weltwirschaftliches Archiv, 132(4), pp. 638–650.
Borland, J., and S. Kennedy (1998), ‘Dimensions, Structure and History of Australian Unemployment’, paper presented at this conference.
Bradbury, B. (1995), ‘Added, Subtracted or Just Different: Why do the Wives of Unemployed Men Have Such Low Employment Rates’, Australian Bulletin of Labour, 21(1), pp. 48–70.
Breusch, T. (1978), ‘Testing for Autocorrelation in Dynamic Linear Models’, Australian Economic Papers, 17(31), pp. 334–355.
Brooker, R. (1993), ‘Structural Modelling of Unemployment’, paper presented at the Access Economics, Centre for Economic Policy Research National Forecasting and Economic Policy Conference.
Burgess, S. (1993), ‘Labour Demand, Quantity Constraints or Matching’, European Economic Review, 37(7), pp. 1295–1314.
Caballero, R. and M. Hammour (1996), ‘On the Timing and Efficiency of Creative Destruction’, Quarterly Journal of Economics, 111(3), pp. 805–852.
Chapman, B. (1990), ‘The Labour Market’, in S. Grenville (ed.), The Australian Macroeconomy in the 1980s, Reserve Bank of Australia, Sydney, pp. 7–65.
Chapman, B. and F. Gruen (1990), ‘An Analysis of the Australian Consensual Incomes Policy: The Prices and Incomes Accord’, Australian National University Centre for Economic Policy Research Discussion Paper No. 221.
Cockerell, L. and B. Russell (1995), ‘Australian Wage and Price Inflation: 1971–1994’, Reserve Bank of Australia Research Discussion Paper No. 9509.
Commonwealth Treasury (1996), User's Guide to the TRYM Model of the Australian Economy, CPN Printing, Canberra.
Connolly, G. and K. Spence (1996), ‘The Role of Consumer Loan Affordability and Other Factors Influencing Family Decisions in Determining the Female Full-time Participation Rate in the Australian Labour Force’, paper presented at the 25th Conference of Economists, Australian National University, Canberra.
Crosby, M. and N. Olekalns (1998), ‘Inflation, Unemployment and the NAIRU in Australia’, Australian Economic Review, forthcoming.
Debelle G. and J. Vickery (1997), ‘Is the Phillips Curve a Curve? Some Evidence and Implications for Australia’, Reserve Bank of Australia Research Discussion Paper No. 9706.
Dixon, R. (1996), ‘Apparent Asymmetries in the Relationship Between the Participation Rate and the Employment Rate in Australia’, University of Melbourne Department of Economics Research Paper No. 522.
Downes, P. and G. Stacey (1996), ‘The NAIRU in the Treasury Macroeconomic (TRYM) Model of the Australian Economy: Definition, Measurement and Policy Implications’, Note Prepared for Input into the OECD, Economic Policy Committee Working Party 1, Programme of Work, Commonwealth Treasury, Canberra, <http://www.treasury.gov.au/publications/TRYM>.
Dowrick, S. (1988), ‘Labour Supply Trends and Prospects for Unemployment in the Medium Term’, Australian National University Centre for Economic Policy Research Discussion Paper No. 188.
Dungey, M. and J. Pitchford (1998), ‘Prospects for Output and Employment Growth with Steady Inflation’, paper presented at this conference.
Dunlop, Y., T. Healy and P. McMahon (1984), ‘Australian Models of Labour Force Participation: A Critical Review’, in A. Kaspura (ed.), Labour Force Participation in Australia, The Proceedings of a Conference, Bureau of Labour Market Research, Australian Government Printing Service, Canberra, pp. 17–39.
Elmsekov, J. and K. Pichelmann (1993), ‘Unemployment and Labour Force Participation – Trends and Cycles’, OECD Economics Department Working Paper No. 130.
Fahrer, J. and A. Pease (1993), ‘The Unemployment/Vacancy Relationship in Australia’, Australian Economic Review, 26(4), pp. 43–57.
Gregory, R. (1991), ‘Jobs and Gender: A Lego Approach to the Australian Labour Market’, Economic Record, 67(supplement), pp. 20–40.
Gregory, R. and R. Duncan (1979), ‘The Labour Market in the 1970s’, in W. Norton (ed.), Conference on Applied Economic Research, Reserve Bank of Australia, Sydney, pp. 256–319.
Gregory, R., P. McMahon and B. Whittingham (1985), ‘Women in the Australian Labour Force: Trends, Causes and Consequences’, Journal of Labor Economics, 3(1), Part 2, pp. S293–S309.
Gruen, D., J. Romalis and N. Chandra (1997), ‘The Lags of Monetary Policy’, Reserve Bank of Australia Research Discussion Paper No. 9702.
Gruen, D. and G. Shuetrim (1994), ‘Internationalisation and the Macroeconomy’, in P. Lowe and J. Dwyer (eds), International Integration of the Australian Economy, Reserve Bank of Australia, Sydney, pp. 309–363.
Hamermesh, D. (1993), Labor Demand, Princeton University Press, Princeton, New Jersey.
Harper, I. (1980), ‘Unemployment and Vacancies in Australia’, Economic Record, 56(154), pp. 231–243.
Hausman, J. (1983), ‘Specification and Estimation of Simultaneous Equation Models’, in Z. Griliches and M. Intriligator (eds), Handbook of Econometrics, North-Holland, Amsterdam, Vol. 1, pp. 392–446.
Indecs (1986), State of Play 4, Allen and Unwin, North Sydney.
Kenyon, P. and M. Wooden (1995), ‘Labour Supply’, in K. Norris and M. Wooden (eds), The Changing Australian Labour Market, Economic Planning Advisory Commission Paper No. 11, AGPS, Canberra, pp. 15–38.
Killingsworth, M. (1983), Labor Supply, Cambridge University Press, Cambridge, England.
Killingsworth, M. and J. Heckman (1986), ‘Female Labor Supply’, in O. Ashenfelter and R. Layard (eds), Handbook of Labor Economics, North-Holland, Amsterdam, Vol. 1, pp. 103–204.
Kremers, J., N. Ericsson and J. Dolado (1992), ‘The Power of Cointegration Tests’, Oxford Bulletin of Economics and Statistics, 54(3), pp. 325–348.
Layard, R. and S. Nickell (1986), ‘Unemployment in Britain’, Economica, 53(210S), pp. S121–S169.
Lewis, P. and M. Kirby (1988), ‘A New Approach to Modelling the Effects of Incomes Policies’, Economics Letters, 28(1), pp. 81–85.
Lilien, D. (1982), ‘Sectoral Shifts and Cyclical Unemployment’, Journal of Political Economy, 90(4), pp. 777–793.
Lindbeck, A. and D. Snower (1988), The Insider-Outsider Theory of Employment and Unemployment, MIT Press, Cambridge, Massachusetts.
Macfarlane, I. (1997), ‘Monetary Policy, Growth and Unemployment’, Reserve Bank of Australia Bulletin, June, pp. 1–8.
Murphy, C. (1992), Access Economics Murphy Model Manual Version 2.1, The Model in Detail, Access Economics Pty Ltd, Canberra.
OECD (1996), OECD Economic Surveys, Australia, OECD, Paris.
Parkin, M. (1973), ‘The Short-run and Long-run Trade-offs between Inflation and Unemployment in Australia’, Australian Economic Papers, 12(21), pp. 127–144.
Pencavel, J. (1986), ‘Labor Supply of Men: A Survey’, in O. Ashenfelter and R. Layard (eds), Handbook of Labour Economics, North-Holland, Amsterdam, Vol. 1, pp. 2–102.
Pissarides, C. (1991), ‘Real Wages and Unemployment in Australia’, Economica, 58(229), pp. 35–55.
Quandt, R. and H. Rosen (1989), ‘Endogenous Output in an Aggregate Model of the Labor Market’, Review of Economics and Statistics, 71(3), pp. 394–400.
Russell, B. and W. Tease (1991), ‘Employment, Output and Real Wages’, Economic Record, 67(196), pp. 34–45.
Sargent, T. (1978), ‘Estimation of Dynamic Labor Demand Schedules under Rational Expectations’, Journal of Political Economy, 86(6), pp. 1009–1044.
Shapiro, C. and J. Stiglitz (1984), ‘Equilibrium Unemployment as a Worker-Discipline Device’, American Economic Review, 74(2), pp. 433–444.
Simes, R. and C. Richardson (1987), ‘Wage Determination in Australia’, Economic Record, 63(181), pp. 144–155.
Stacey, G. and P. Downes (1995), ‘Wage Determination and the Labour Market in the Treasury Macroeconomic (TRYM) Model’, paper presented at the 24th Conference of Economists, Adelaide.
Watts, M. and W. Mitchell (1990), ‘Australian Wage Inflation: Real Wage Resistance, Hysteresis and Incomes Policy: 1968(3)–1988(3)’, The Manchester School, 58(2), pp. 142–164.